Geometrical Methods in Recent Music Theory
Dmitri Tymoczko
REFERENCE: http://www.mtosmt.org/issues/mto.09.15.5/mto.09.15.5.rockwell.html
Copyright © 2010 Society for Music Theory
[1] The following remarks are prompted by Joti Rockwell’s interesting article, “Birdcage Flights: A Perspective on Inter-Cardinality Voice Leading” (2009). My goal is not to take issue with Rockwell’s specific claims but rather to underscore a few details that might escape the casual reader’s attention. In particular, I want to stress three basic points.
- Rockwell’s discrete “birdcage graphs,” which represent efficient voice leadings between chords of different sizes, can be embedded within the infinite-dimensional “OPC space” discussed by Callender, Quinn, and myself.(1) In general, discrete voice-leading graphs can always be embedded within the continuous spaces we describe.
- Because of this, Rockwell’s graphs inherit some of the complications of C space, and may distort voice-leading relationships among nonadjacent chords.
- This is actually symptomatic of a more general issue affecting a variety of music-theoretical graphs. Roughly speaking, we have no guarantee that graphs whose edges refer to musical motions of a particular type will give rise to an intuitive or familiar notion of “distance” between nonadjacent chords.
[2] This last item is significant because theorists sometimes seem to endorse the following methodology. First, one selects some interesting domain of musical objects and some interesting set of motions among them. (For example, single-voice voice-leading between major and minor triads.) Second, one constructs a graph representing all of these motions between all the objects in question. Third, one interprets the resulting graph as providing a measure of distance. Thus, for example, one might use the graph to analyze music that moves between non-adjacent chords, or claim that larger leaps on the graph are musically disfavored in some way.
[3] This last step, however, involves a subtle leap. Consider, for example, the familiar Tonnetz (Figure 1).(2) Two chords are adjacent on this graph if they can be linked by what Cohn calls “parsimonious voice leading”: voice leading in which a single voice moves, and it moves by just one or two semitones (Cohn 1996). However, larger distances in the space do not faithfully mirror voice-leading facts. On the Tonnetz, C major is two units away from F major but three units from F minor—even though it takes just two semitones of total motion to move from C major to F minor, and three to move from C major to F major (Figure 2). (This is precisely why F minor so often appears as a passing chord between F major and C major.) It follows that we cannot use the Tonnetz to explain the ubiquitous nineteenth-century IV-iv-I progression, in which the two-semitone motion ![]() →
→![]() is broken into the semitonal steps
is broken into the semitonal steps ![]() →
→![]()
![]() →
→![]() . More generally, it shows that Tonnetz-distances do not correspond to voice-leading distances in any straightforward way (Tymoczko 2009).
. More generally, it shows that Tonnetz-distances do not correspond to voice-leading distances in any straightforward way (Tymoczko 2009).
|
Figure 1. The Tonnetz (click to enlarge) |
Figure 2 (click to enlarge and see the rest) |
[4] Note that the problem persists even if we try to reinterpret the Tonnetz as representing common tones rather than voice leading: both F minor and E![]() minor are three Tonnetz-steps away from C major, even though C major and F minor have one common tone, while C major and E
minor are three Tonnetz-steps away from C major, even though C major and F minor have one common tone, while C major and E![]() minor have none. (As before, shorter distances are easier to interpret: two chords are adjacent on the Tonnetz if they have two common tones, and any pair of chords that are two steps away will share exactly one common tone.) Thus, neither voice leading nor common tones allow us to characterize Tonnetz distances precisely. We seem forced to say that Tonnetz-distances represent simply the number of parsimonious
moves needed to get from one chord to another—and not some more familiar music-theoretical quality.
minor have none. (As before, shorter distances are easier to interpret: two chords are adjacent on the Tonnetz if they have two common tones, and any pair of chords that are two steps away will share exactly one common tone.) Thus, neither voice leading nor common tones allow us to characterize Tonnetz distances precisely. We seem forced to say that Tonnetz-distances represent simply the number of parsimonious
moves needed to get from one chord to another—and not some more familiar music-theoretical quality.
[5] From this point of view, there is a fundamental difference between the Tonnetz and Douthett and Steinbach’s “Cube Dance” (Douthett and Steinbach 1998). (Figure 3) Like the Tonnetz, “Cube Dance” depicts a collection of local moves, in this case the single-semitone voice leadings between major, minor, and augmented triads. (On Douthett and Steinbach’s graph, descending semitonal motion is represented by clockwise steps, while ascending semitonal motion is represented by counterclockwise steps.) Unlike the Tonnetz, however, “Cube Dance” also faithfully models voice-leading distances between non-adjacent chords; in fact, any clockwise or counterclockwise path on “Cube Dance” can be associated with a particular voice leading, with the length of the path (as measured in edges) corresponding to the size of the voice leading (as measured according to “taxicab distance,” or the total number of semitonal steps in all voices). Compared to the Tonnetz, then, “Cube Dance” is a more genuinely geometrical, modeling musically familiar distances between nonadjacent objects. To be sure, this distinction may not be intuitively obvious on first inspection. In fact, the difference between these two sorts of graphs only became clear after theorists discovered how to construct the n-dimensional spaces representing all possible n-voice voice-leadings between all possible n-note chords.(3)
|
Figure 3. Douthett and Steinbach’s “Cube Dance” (click to enlarge and see the rest) |
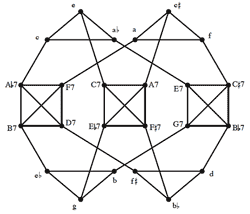
Figure 4. Rockwell’s “Birdcage Graph” (click to enlarge) |
[6] With this distinction in mind, let’s now turn to Rockwell’s “birdcage graphs.” Figure 4, which is reproduced from Rockwell’s article, connects dominant seventh chords and minor triads if they can be linked by voice leading in which two voices move by semitone. Thus A![]() 7 and C minor are adjacent because they can be linked the “augmented sixth” voice leading (A
7 and C minor are adjacent because they can be linked the “augmented sixth” voice leading (A![]() , C, E
, C, E![]() , G
, G![]() )→(G, C, E
)→(G, C, E![]() , G), and C minor and A
, G), and C minor and A![]() minor are adjacent because they can be connected by the voice leading (C, E
minor are adjacent because they can be connected by the voice leading (C, E![]() , G)→(C
, G)→(C![]() , E
, E![]() , A
, A![]() ). But notice that the larger distances are not so easy to interpret: A
). But notice that the larger distances are not so easy to interpret: A![]() 7 and C7 are both equidistant from A
7 and C7 are both equidistant from A![]() minor, even though the minimal voice leading from A
minor, even though the minimal voice leading from A![]() 7 to A
7 to A![]() minor, (A
minor, (A![]() , C, E
, C, E![]() , G
, G![]() )→(A
)→(A![]() , C
, C![]() , E
, E![]() , A
, A![]() ), involves three semitones of total motion, while the minimal voice leading from C7 to A
), involves three semitones of total motion, while the minimal voice leading from C7 to A![]() minor, (C, E, G, B
minor, (C, E, G, B![]() )→(C
)→(C![]() , E
, E![]() , A
, A![]() , C
, C![]() ), involves four. Thus, though single-edge motions on Rockwell’s graph refer to a particular sort of voice leading (single-semitone motion in two distinct voices), the two-edge
motions do not. Figure 5 shows that this is because there are various ways to combine the graph’s voice leadings: in Figure 5(a) the two motions in the bass cancel out, while in Figure 5(b) no such cancellation occurs.
), involves four. Thus, though single-edge motions on Rockwell’s graph refer to a particular sort of voice leading (single-semitone motion in two distinct voices), the two-edge
motions do not. Figure 5 shows that this is because there are various ways to combine the graph’s voice leadings: in Figure 5(a) the two motions in the bass cancel out, while in Figure 5(b) no such cancellation occurs.
Figure 5. The voice leadings on Rockwell’s graph can be combined in multiple nonequivalent ways
(click to enlarge)
Figure 6
(click to enlarge)
[7] The problem here is symptomatic of a larger issue, namely that it is difficult to represent voice-leading relations between chords of different sizes. Importantly, this is as true for discrete graphs as it is for “C space” in all its infinite-dimensional glory. For another example, consider Figure 6, which faithfully depicts single-semitone voice leadings between chromatic clusters, semitones, and single notes. (On this graph, two chords are adjacent if they can be linked by a voice-leading in which some notes are doubled, and in which one voice moves by one semitone.)
However, the larger distances again diverge from voice leading distances: the graph depicts {F![]() , G, A
, G, A![]() } (=678) and {C
} (=678) and {C![]() , D, E
, D, E![]() } (=123) as being seven edges away from one another, even though the minimal voice leading between them involves at least fifteen total semitones of motion.(4) A central conclusion of Callender, Quinn, and Tymoczko (2008) is that similar problems will inevitably appear as we try to subsume more and more chords (of differing sizes) within our graphs: to obtain completeness without sacrificing contrapuntal fidelity, we must restrict ourselves to multisets of some particular size.
} (=123) as being seven edges away from one another, even though the minimal voice leading between them involves at least fifteen total semitones of motion.(4) A central conclusion of Callender, Quinn, and Tymoczko (2008) is that similar problems will inevitably appear as we try to subsume more and more chords (of differing sizes) within our graphs: to obtain completeness without sacrificing contrapuntal fidelity, we must restrict ourselves to multisets of some particular size.
[8] The broader moral is that we should take care to distinguish two different sorts of music-theoretical models. The first represents only a collection of local relationships: at any point in the space, it shows us all the available “moves” of a certain kind. (Here we might think of a subway map that shows which stations are adjacent to any other station.) The second type may do this as well, but it also captures some familiar notion of distance between all the objects it represents—even those that are not immediately adjacent on the graph. The important point is that we have no guarantee that any particular notion of musical distance will necessarily give rise to any coherent geometry of this second sort. (It is, for example, quite difficult to construct a geometrical space whose points represent major and minor triads, and in which distance represents the number of common tones.) Nor, conversely, can we be sure that a particular collection of local moves give rise to a familiar notion of musical distance. From this point of view, the remarkable fact is that we can construct coherent geometries in the special case where we are concerned with voice leading among multisets of a fixed size.
[9] Of course, none of this is meant as a criticism of Rockwell, or as an objection to his useful graphical constructions. My point, rather, is that we need caution when interpreting the sorts of structures he describes. Some graphs are useful primarily insofar as they depict a collection of local moves, while others give rise to a more complex geometry, and the difference may not always apparent upon casual inspection.
Dmitri Tymoczko
Princeton University
dmitri@princeton.edu
Works Cited
Callender, Clifton, Ian Quinn, and Dmitri Tymoczko. 2008. “Generalized Voice Leading Spaces,” Science 320: 346–348.
Cohn, Richard. 1996. “Maximally Smooth Cycles, Hexatonic Systems, and the Analysis of Late-Romantic Triadic Progressions.” Music Analysis 15.1: 9–40.
Douthett, Jack and Peter Steinbach. 1998. “Parsimonious Graphs: a Study in Parsimony, Contextual Transformations, and Modes of Limited Transposition.” Journal of Music Theory 42.2: 241–263.
Hyer, Brian. 2002. “Tonality.” In The Cambridge History of Western Music Theory, ed. Thomas Christensen. New York: Cambridge University Press.
Mooney, Kevin. 1996. “The ‘Table of Relations’ and Music Psychology in Hugo Riemann’s Harmonic Theory.” Ph. D. Thesis, Columbia University.
Rockwell, Joti. 2009. “Birdcage Flights: A Perspective on Inter-Cardinality Voice Leading.” Music Theory Online 15.5.
Tymoczko, Dmitri. 2004. “Scale Networks in Debussy.” Journal of Music Theory 48.2: 215–292.
—————. 2006. “The Geometry of Musical Chords.” Science 313: 72–74.
—————. 2008. “Scale Theory, Serial Theory, and Voice Leading.” Music Analysis 27.1: 1–49.
—————. 2009. “Three Conceptions of Musical Distance.” In Mathematics and Computation in Music, eds. Elaine Chew, Adrian Childs, and Ching-Hua Chuan, Heidelberg: Springer, 258–273.
—————. 2010. A Geometry of Music. New York: Oxford.
Footnotes
1. OPC space is the geometrical space that is formed when we discard the octave, order, and multiplicity of groups of notes. From this point of view (C4, E4, G4) is equivalent to (E3, G4, G5, C2, E4). The space is infinite dimensional because it contains sequences of arbitrary length. See Callender, Quinn, and Tymoczko 2008.
Return to text
2. See Mooney 1996, Hyer 2002, and Cohn 1996 for the discussion of the Tonnetz and its history.
Return to text
3. See Tymoczko 2006, 2009 and 2010, the last of which shows that both Douthett and Steinbach’s “Power Towers” and my own “scale lattice” (Tymoczko 2004) faithfully reflect voice-leading distances between non-adjacent chords.
Return to text
4. The issue here is that the shortest graphical path between {F![]() , G, A
, G, A![]() } and {C
} and {C![]() , D, E
, D, E![]() } involves a series of voice leadings with different numbers of voices: one can collapse the three-note {F
} involves a series of voice leadings with different numbers of voices: one can collapse the three-note {F![]() , G, A
, G, A![]() } onto {F
} onto {F![]() , G} by a single edge, representing the three-voice voice leading (F
, G} by a single edge, representing the three-voice voice leading (F![]() , G, A
, G, A![]() )→(F
)→(F![]() , G, G); {F
, G, G); {F![]() , G} can then be collapsed to F
, G} can then be collapsed to F![]() by an edge that represents the two-voice voice leading (F
by an edge that represents the two-voice voice leading (F![]() , G)→(F
, G)→(F![]() , F
, F![]() ). Moving F
). Moving F![]() to E
to E![]() then takes three more edges, representing the one-voice voice-leading F
then takes three more edges, representing the one-voice voice-leading F![]() →E
→E![]() . (This E
. (This E![]() then expands to {C
then expands to {C![]() , D, E
, D, E![]() } by two more edges representing two- and three-voice voice leadings.) Since these component voice leadings have different numbers of voices, they do not combine to form a three-voice voice leading in which the voices move by a collective total of seven semitones. This is just a discrete version of the example discussed in Callender, Quinn, and Tymoczko (2008), supplementary section 4.
} by two more edges representing two- and three-voice voice leadings.) Since these component voice leadings have different numbers of voices, they do not combine to form a three-voice voice leading in which the voices move by a collective total of seven semitones. This is just a discrete version of the example discussed in Callender, Quinn, and Tymoczko (2008), supplementary section 4.
Return to text
Copyright Statement
Copyright © 2010 by the Society for Music Theory. All rights reserved.
[1] Copyrights for individual items published in Music Theory Online (MTO) are held by their authors. Items appearing in MTO may be saved and stored in electronic or paper form, and may be shared among individuals for purposes of scholarly research or discussion, but may not be republished in any form, electronic or print, without prior, written permission from the author(s), and advance notification of the editors of MTO.
[2] Any redistributed form of items published in MTO must include the following information in a form appropriate to the medium in which the items are to appear:
This item appeared in Music Theory Online in [VOLUME #, ISSUE #] on [DAY/MONTH/YEAR]. It was authored by [FULL NAME, EMAIL ADDRESS], with whose written permission it is reprinted here.
[3] Libraries may archive issues of MTO in electronic or paper form for public access so long as each issue is stored in its entirety, and no access fee is charged. Exceptions to these requirements must be approved in writing by the editors of MTO, who will act in accordance with the decisions of the Society for Music Theory.
This document and all portions thereof are protected by U.S. and international copyright laws. Material contained herein may be copied and/or distributed for research purposes only.
Prepared by Sean Atkinson, Editorial Assistant