Re: Eytan Agmon on Functional Theory

[1] Eytan Agmon’s recent article “Functional Harmony Revisited”⁽¹⁾proposes a theory of functional meaning for harmonic degrees related to that of Hugo Riemann but differing from it by virtue of “greater theoretical rigor and the removal of arbitrary features” (211). As Agmon explains, “the hallmarks of functionalism are: (1) the characterization of individual chords as tonic (T), subdominant (S), or dominant (D) in function; and (2) the notion that the so-called primary triads, I, IV, and V somehow embody the essence of each of these functional categories.” These are the characteristics of functionalism that Agmon wishes to preserve.⁽²⁾

[2] Agmon begins by situating functional theory in a larger intellectual context, presenting it as, in effect, a special case of what is known as prototype theory: “Indeed, one way of stating the core idea of the present article is: given a separation of chord progression from harmonic function, the notions function and primary triad are fully reducible to category and prototype, respectively” (199).(3) Note well what is stated here as a “given”: harmonic function is conceived as entirely separable from chord progression. We shall return to this presupposition shortly.

[3] Agmon’s theory is admirable in its simplicity. Its principal components are (1) a definition, based on note-content, of degree of triadic similarity for diatonic triads; (2) the specification of three “principles” that “uniquely select the triads I, IV, and V as prototypes of three harmonic categories. . .” (201); and (3) two additional “principles” that “determine the additional members of each category and their respective prototypicalities.” Of the five “principles,” two that select prototypes and one that selects additional members are described as “self-evident,” a status that might be granted one of the other two as well—namely the principle that prototypes must not be maximally similar to each other.⁽⁴⁾ The remaining principle, however,—the principle of symmetry, which states that the graphic symmetry of Agmon’s Fig. 2c, quoted here as example 1, “must not be violated”—is not self-evident, nor is its necessity established by Agmon on any persuasive independent basis.

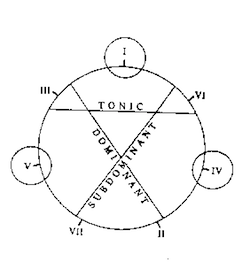
[4] The diagram in Example 1 shows the harmony of II as standing within the subdominant function, but also, just slightly, within the dominant. Agmon puts this into words at a later point in his essay with the following assertion: “although the function of II is primarily subdominant, a weak dominant function nevertheless exists” (206). Does this mean that II can be both subdominant and dominant at the same time? Apparently not: “. . .the dominant function of II, I believe, may be felt in certain contexts where II (or II⁶) is followed by I (or I⁶).” Thus the previously “given” separation of chord function from harmonic progression is, at least in this instance, retracted. As we shall see later, it must be retracted not in this one instance only, but, indeed, across the board.

[5] Probably the central core of functional theory (the part that Agmon wishes to retain), unlike many of Riemann’s ideas, was not merely a case of “theory for the sake of theory,” but was rather a well-meaning attempt to respond to problems posed by a wide variety of perceptual phenomena. Let us examine one such phenomenon in detail. Consider the final cadence of Schumann’s “Am Kamin” from Kinderszenen, shown in Example 2. The three-note penultimate chord c–e–a contains the notes of a III in F major, but has the “aura” or in Agmon’s term the “essence” of the dominant; this elusive “aura” is, I suspect, what is sought to be represented by the word “function” in functional theory, most of whose practitioners would here assign the symbol D for dominant. Henceforth in this review I shall (in most cases) enclose in quotation marks any Roman numeral that represents literal pitch content but not “aura,” which latter entity will be designated by Roman numeral without quotation marks. Thus in Schumann’s cadence, the “III” means V.

[6] Why does this “III” mean V? At least two reasons can be adduced from the structure of Schumann’s phrase, which is displayed in Example 3. First, and probably most important, the treble voice negotiates a fifth-progression (a re-drawing, here in the coda, of the Urlinie descent). The last passing tone in that fifth, the g of the penultimate bar, moves to f at the end; the note a that intervenes, far from obliterating the g, takes on a subordinate role as a kind of embellishment or enhancement of the fundamental stepwise progression g–f. To be exact, it serves as what is variously termed an escape tone or an incomplete upper neighbor, but is perhaps still more precisely understood as an anticipation of the third of the coming tonic harmony. In any case, g implicitly but effectively remains present as the fifth of c. The third- space delineated by the succession a–f associates weakly with the preceding one from b♭ to g (see the brackets).

[7] Secondly, an independent force is at work here, one that may as well be called harmonic syntax, or the syntax of scale- degree progression. The simplified harmonic basis of the passage is shown in Example 4a.

[8] We may perhaps agree with functional theory that “the so-called primary triads, I, IV, and V” are indeed primary in some meaningful sense. Example 4a is based on the succession of these primary harmonies prescribed by the most fundamental principle of their syntax: that of progression by fifth.⁽⁵⁾ (Example 4b explains the origin of Schumann’s II as the result of extending the bass of IV and letting the treble anticipate the fifth of the coming V.) The implication of the first falling fifth, f–b♭, which could cast doubt on the identity of the tonic, is set right by the second one, c–f. Given the construction of the bass, people who hear musically will have a strong predilection to hear this final fifth as representing V–I even though its penultimate member does not bear the sonority which alone would provide full congruence between scale-degree meaning and vertical chord. Add to this predilection the melodic factors described above and the penultimate chord c–e–a is heard as unmistakably expressing the “aura” of the dominant. It is a harmonic realization of the dominant scale degree. This means that the note a in no sense functions as a harmonic root here. The chord under discussion is not an inversion of an a-minor triad. Although it contains the notes of the triad of the mediant, if the Roman numeral as an analytic symbol is to reflect aural qualities of the music as heard by a perceptive listener rather than merely the appearance of the notation, the Roman numeral III cannot, without the accompanying “shudder-quotes” I have used here, accurately be applied to it.

[9] When I say that in this case “III” means V, the word means may be explicated as “constitutes, or is included within, a harmonic expression of.” “III” may equally well mean I; “II” may mean IV; indeed, instances of “X” meaning Y are legion in the repertoire of tonal music, and virtually no a- priori limits can be set on the ranges of ‘X’ and ‘Y’.⁽⁶⁾

[10] This peculiarity of the relationship of momentary note- content to harmonic entities was grasped, to a large extent, by Heinrich Schenker as early as 1906: “. . . not every triad must be considered as a scale-step. . . .”⁽⁷⁾ Schenker’s subsequent work might be construed as a massive effort to explicate this perception, whose most concise and complete modern verbal formulation has been provided by Carl Schachter: “There is no such ‘thing’ as a I chord in C major, but only an idea that can find expression through the notes C, E, and G in any kind of simultaneous blending, through intervals created by two of these notes, through the note C alone, through such combinations as C–E♭–G, C–E–G–A, and C–E–G–B♭, through melodic lines of the most various shapes, through whole constellations of contrapuntal lines and chord successions controlled by the note C.”⁽⁸⁾ This improves on Schenker’s clairvoyant but underexplicit 1906 formulation in its recognition that the relation between vertical note-combination and scale degree is even far less intimate than Schenker’s early statement might suggest: altogether, it is not a note-combination but an “idea” (or “aura” or “essence”) that is designated by the properly applied Roman numeral.

[11] Unfortunately, however, this is not what Roman numeral and scale degree mean to Agmon. About Brahms’s Intermezzo Op. 117, No. 2, he writes that “. . . in the consequent phrase, which begins in measure 9, the opening II6 chord concludes the dominant prolongation which begins in measure 6 . . .” (208). The chord referred to is the last chord of bar 9, and it does indeed occur within a prolongation of the dominant.⁽⁹⁾To call it II⁶—merely to use the Roman numeral in this way—is to devalue a profoundly meaningful analytic symbol by turning it into a mere mechanical reduction of a trivial transliteration of note-content. Agmon, it is clear, speaks the language of the completely conventional harmony textbook.

[12] The theory of tonal music is thus effectively deprived of the scale degree in Schenker’s visionary conception of it. Once the scale degree has been so devalued to identity with vertical note-content, as it invariably is in Agmon’s article, the inevitable, and completely unacceptable, result is a theoretical void.⁽¹⁰⁾ There is simply no longer any available theoretical correlate for certain palpable musical realities.

[13] In certain contexts, function-theoretic analysis fills this void in a way that is perhaps not objectionable. Agmon’s example 4a is given here as Example 5. It is quoted by Agmon from Aldwell and Schachter’s harmony textbook,⁽¹¹⁾ with only the replacement of the latter’s (completely sufficient) “IV–I” by “S–T.” In such a case, functional theory could be regarded as relatively benign. There would be no substantive objection to the replacement of the symbols; after all, “IV” and “subdominant” are interchangeable for almost all purposes. For its raison d’etre, however, functional theory would still be indebted only to the trivialization of scale degree and Roman numeral just described.

[14] Functional theory goes much further, though, because it insists that (for example) II always represents one of the primary categories—usually S, less often D (see example 1). How plausible is this claim when it is applied to actual music—for example, to the opening bars of Haydn’s piano sonata Hob. 52, Finale (see Example 6)? The music of bar 1ff. composes out the tonic scale degree, the I. Beginning at the end of bar 8, the same diminution is applied to the second scale degree, the II. Clearly, Haydn has moved up a step. What is to be gained by insisting that the resulting F-minor area stands for anything but scale degree II—by claiming that it represents, for example, the subdominant? The justification for such a claim might argue from the fact that this harmony, like the subdominant so often, moves to V; if so, then all pretense of treating function apart from progression would have to be renounced.⁽¹²⁾ In some cases— chiefly when $\hat{2}$ appears in an inner voice, which is hardly the case here—it might be said that II “sounds” (somewhat) like IV (at least to most undergraduate students); this by no means justifies depriving II of its independent place as a harmony in the key.

[15] Worse still, to assert that this II “is” a version of the subdominant would lead to certain bizarre results. It would mean, for example, that the relation between the root of the F-minor harmony in bar 9ff. of example 6 and the root of the initial tonic is primarily to be understood as a fifth rather than a second; and moreover, that the harmonic progression in bars 16–17 is by second rather than by fifth. This is where functional theory ceases to be benign and becomes pernicious.

[16] Thus “II” need not represent IV. It may do so, of course, as has long been understood. In case it does, the explanation is to be sought in domains other than harmonic theory. A careful consideration of such a “II” in its context will show that its constituent scale degree $\hat{2}$has a linear mission (e.g. as a passing or neighboring note).⁽¹³⁾Such a mission excludes any interpretation of $\hat{2}$ as a harmonic root, and thus excludes an interpretation of such a “II” as II.⁽¹⁴⁾

[17] Agmon had no choice, therefore, but to retract (in at least one instance) his postulate that “harmonic function” can be treated apart from “chord progression.” He represents the case in which he does retract it as special, but in fact the circumstances that lead him to take “chord progression” into consideration there are completely general and equally present everywhere in music. And it is not merely “chord progression” but voice leading, meter and rhythm, motif, and in brief all aspects of what Schenker called Auskomponierung that must be considered in assessing the function—harmonic and otherwise—of chordal entities as they occur in music. Functional theory remains a superfluous appendage so long as we do not discard what has been learned about music thus far.

John Rothgeb
Binghamton University
Department of Music
Binghamton, NY 13902-6000
rothgeb@bingsuns.cc.binghamton.edu

Footnotes

1. Music Theory Spectrum 17/2 (Fall, 1995), 196–214.
Return to text

2. Among the features he is willing to abandon is Riemann’s notion of Scheinkonsonanz, the idea that secondary triads are merely “apparent” consonances, each being accompanied always, if only tacitly, by an associated “characteristic dissonance.”
Return to text

3. Later (202), by analogy, “functional strength” is said to reduce to “prototypicality.”
Return to text

4. Agmon puts this too strongly in his statement that “prototypes must be maximally dissimilar to each other . . .” (201). I and IV, for example, are both prototypes, but their degree of similarity by his measure is “intermediate” rather than “minimal”; this must surely entail that their degree of dissimilarity is less than maximal.
Return to text

5. Schenker occasionally speaks evocatively of the “Quintengeist der Stufen.” Agmon acknowledges “the privileged status of certain root relationships, most notably by descending fifth” (211).
Return to text

6. Certain limits would probably stand up under scrutiny. Although “II” can, under certain circumstances, be the sole constituent of an expression of IV, it is doubtful that “I” and V, for example, could be so related.
Return to text

7. Heinrich Schenker, Harmony (ed. O. Jonas, trans. E.M. Borghese; Chicago: University of Chicago Press, 1954), 139.
Return to text

8. Schachter, “Either/Or,” in H. Siegel, ed, Schenker Studies (Cambridge: Cambridge University Press, 1990), 166.
Return to text

9. The identical chord in the upbeat to bar 1 harmonizes a passing tone within a tonic prolongation. Agmon applies to it as well the functional symbol D — surely a gross overburdening with harmonic significance of one of the most elemental of contrapuntal phenomena: parallel motion in chords.
Return to text

10. Riemann, of course, had to be completely innocent of Schenker’s breakthrough, and thus he cannot be accused of having trivialized an earlier important theoretical insight. Today matters are different, and it is discouraging that the best insights in our discipline remain incompletely understood.
Return to text

11. Edward Aldwell and Carl Schachter, Harmony and Voice Leading (second edition, New York: 1989), 392.
Return to text

12. For all that the similar behavior of II and IV is conventional wisdom, analytic theory and analytic insight in fact gain nothing by its affirmation, which is merely a compromise convenient for certain pedagogical purposes.
Return to text

13. Here I draw attention particularly to Agmon’s statement, quoted earlier, that “. . .the dominant function of II. . . may be felt in certain contexts. . .” (emphasis added). It is not only “II”, however, but every chord whose meaning is ascertainable only with reference to all features of its context.
Return to text

14. It does not, however, exclude the possibilty that IV may appear to “turn into” II in the course of prolongation. Such a reinterpretation exploits the equivocality of the chord, in which the 6 may in principle represent either a linear element or the root of an inverted chord. When such a 6 is so reinterpreted as a root, it may be reincarnated as the bass of a . See Schenker, Free Composition (trans. E. Oster; New York: Longman, 1979), p. 90, “Addition of a Root.” Much complexity is added to this topic (a full treatment of which exceeds the scope of this review) by the relation between structural levels: a note that arises in the background by a linear process may become a “root” in the foreground. This is fully analogous to the notion of “key areas” as foreground “illusions.”
Return to text

The identical chord in the upbeat to bar 1 harmonizes a passing tone within a tonic prolongation. Agmon applies to it as well the functional symbol D — surely a gross overburdening with harmonic significance of one of the most elemental of contrapuntal phenomena: parallel motion in chords.

It does not, however, exclude the possibilty that IV may appear to “turn into” II in the course of prolongation. Such a reinterpretation exploits the equivocality of the chord, in which the 6 may in principle represent either a linear element or the root of an inverted chord. When such a 6 is so reinterpreted as a root, it may be reincarnated as the bass of a . See Schenker, Free Composition (trans. E. Oster; New York: Longman, 1979), p. 90, “Addition of a Root.” Much complexity is added to this topic (a full treatment of which exceeds the scope of this review) by the relation between structural levels: a note that arises in the background by a linear process may become a “root” in the foreground. This is fully analogous to the notion of “key areas” as foreground “illusions.”

Copyright Statement

Copyright © 1996 by the Society for Music Theory. All rights reserved.

[1] Copyrights for individual items published in Music Theory Online (MTO) are held by their authors. Items appearing in MTO may be saved and stored in electronic or paper form, and may be shared among individuals for purposes of scholarly research or discussion, but may not be republished in any form, electronic or print, without prior, written permission from the author(s), and advance notification of the editors of MTO.

[2] Any redistributed form of items published in MTO must include the following information in a form appropriate to the medium in which the items are to appear:

This item appeared in Music Theory Online in [VOLUME #, ISSUE #] on [DAY/MONTH/YEAR]. It was authored by [FULL NAME, EMAIL ADDRESS], with whose written permission it is reprinted here.

[3] Libraries may archive issues of MTO in electronic or paper form for public access so long as each issue is stored in its entirety, and no access fee is charged. Exceptions to these requirements must be approved in writing by the editors of MTO, who will act in accordance with the decisions of the Society for Music Theory.

This document and all portions thereof are protected by U.S. and international copyright laws. Material contained herein may be copied and/or distributed for research purposes only.