Voice-Leading Transformation and Generative Theories of Tonal Structure
Jason Yust
KEYWORDS: Schenker, tonal structure, voice-leading level, counterpoint, generative theory, generative grammar
ABSTRACT: Numerous generative approaches to explaining tonal structure and/or Schenker’s theories have been proposed since Babbitt noted a resemblance between Schenker’s analytical method and Chomskian generative grammars in 1965. One of the more challenging features of Schenker’s theory to replicate in a generative system is the interaction of counterpoint and hierarchy. Many theorists, such as Lerdahl and Jackendoff, skirt the problem by developing non-contrapuntal systems, meaning ones that do not allow for layers with conflicting hierarchical descriptions. This article tackles the counterpoint problem by first proposing a dynamic model for tonal hierarchy, which matches the usage of basic Schenkerian symbols (slurs and beams), and differs from the representational model used by Lerdahl and Jackendoff and others. I then summarize Schenker’s argument for a contrapuntal theory of tonal structure and show that this implies a relativity of contrapuntal voices to structural level which necessitates a theory of voice-leading transformation. This concept of voice-leading transformation marks a crucial turning point in Schenker’s analytical practice leading directly to his theory of levels, and is fundamental to understanding his late theory. The article also operationalizes the idea of voice-leading transformations within a generative system, and illustrates it with short analyses of themes from Bach’s Partitas and an extended analysis of the Menuetto from Beethoven’s Op. 21 Piano Sonata. In the latter analysis the concept of voice-leading transformation facilitates the discovery of an exceptional feature in the deep middleground of the piece.
Copyright © 2015 Society for Music Theory
[0.1.1] As the theories of Heinrich Schenker were gaining currency in the United States in the mid-twentieth century, Milton Babbitt observed that Schenker’s theory of voice-leading levels was “strikingly similar to transformational grammars in linguistics” (1965, 60). Babbitt’s appraisal of Schenker’s theory inspired the next generation of music theorists, who produced a multiplicity of generative approaches. Many of these were intended as formalizations of Schenker’s method, such as Kassler (1967, 1975), Rahn (1979), and Smoliar (1980). Others were expressly Schenker-inspired, including Keiler (1977), Lerdahl and Jackendoff (1977, 1983), and Westergaard (1975). The generative principle remains a significant feature of how many theorists conceive of Schenkerian analysis, as exemplified by Brown (2005) and Neumeyer (2009).
[0.1.2] Lerdahl and Jackendoff’s Generative Theory of Tonal Music (1983) represents a high-water mark for the first wave of Schenker-inspired generative theories. The widely felt influence of their approach can be attributed in large part to two significant features: First, in observance of the standards of contemporary social science, Lerdahl and Jackendoff strive to be as precise and explicit as possible in the presentation of their theory. This has made their work especially valuable for the empirical study of tonal music,(1) and also led them to make distinctions that might have escaped a looser, more intuitive approach, many of which have shaped subsequent discourse in traditional music theory.(2) Second, Lerdahl and Jackendoff, like other authors cited above, present their work as a theory of tonality, unlike Schenker’s own work, which, as Pastille (1985), Rothstein (1990), Snarrenberg (1997), Cook (2007), and others have pointed out, is about the interpretation of masterworks. This creative misprision of Schenker has a history as old as the Americanization of Schenker itself, and is what allowed Adele Katz (1945) and Felix Salzer (1952), for instance, as well as many others after them, to redirect the theory away from Schenker’s narrow conservative music-historical agenda and draw larger implications from it. Temperley (2011) has advocated reformulating Schenkerian principles as a body of theoretical tenets that can, at least in principle, make empirically verifiable claims.(3)
[0.1.3] This article is organized in four parts. Part 1 reviews generative theories that have been proposed, with special focus on the status of counterpoint, i.e. the structural separability of voices, within them. Parts 2 and 3 operationalize concepts of hierarchy and counterpoint taken from Schenker as an outline of how a generative system derived from his theory might look. Part 2 shows that the hierarchical syntax of Schenkerian symbols is different than the one assumed by most generative approaches. Part 3 discusses the coordination of hierarchy with counterpoint, unpacking a concept of voice-leading transformation that is fundamental to Schenker’s approach. Voice-leading transformations cannot be accommodated by a purely generative system, in loose analogy to the transformational grammar of Chomskian linguistics (the parallel uses of the term “transformation” being, uncannily, fortuitous). Part 4 is an analytical application of the proposed generative system to the Menuetto of Beethoven’s Piano Sonata op. 22, and shows that an explicitly worked out theory of voice-leading levels is needed to appreciate exceptional aspects of the work’s tonal structure.
[0.1.4] The purpose of this exercise is not only to further the development of systematic approaches to describing tonal structure, but also to inform the practice(s) of Schenkerian analysis and even the understanding of Schenker’s theory itself. This effort should, therefore, be of interest to a wide spectrum of music theorists interested in tonal structure, not only those working from a mathematical or computational perspective. This is highlighted in parts 3 and 4 of the paper. The investigation of voice-leading transformation in part 3 reveals important and underappreciated aspects of Schenker’s conception of levels and middleground transformation, and helps clarify the stages in the historical development of his late analytical approach. The application in part four shows how the distinction between generative and transformational operations can clarify what is at stake in specific analytical decisions.
1. Contrapuntal and Non-Contrapuntal Morphologies in Generative Theories
[1.1.1] A generative theory of tonal structure requires at least two components: “morphology” and rules of derivation. Rules of derivation (which can include analytical rules like Lerdahl and Jackendoff’s as well as generative rules) dominate the presentations of most existing theories, yet morphology is arguably more important.
[1.1.2] The idea of tonal morphology is borrowed from linguistics, where it refers to the elemental units of linguistic grammar.(4) The “morphemes” of a theory of tonality are those features that combine to make the “words” of a musical statement, which are musical events. The morphological components of extant generative theories often differ radically. For instance, the objects of Rahn’s (1979) generative system are notes with durational values, while Smoliar’s (1980) and Kassler’s (1967, 1975) objects only have temporal ordering. Sundberg and Lindblöm (1976) and Baroni and Jacoboni (1978, 1983) consider only melodic notes, while other theories incorporate harmony or multiple voices in some way. Even elements of sung verse might be included in a morphology: see the elaborate grammar for Giovanni Legrenzi’s arias proposed by Baroni, Dalmonte, and Jacoboni (2003). Many of these systems derive more abstract properties for musical events, such as functional harmonic designations, for use in the derivational rules. For Lerdahl and Jackendoff (1983), for instance, a musical event consists of a melody note, a Roman-numeral harmonic designation, bass note, and duration. Each of these is a morpheme. On the other hand, Keiler’s (1977) morphology is comparatively simple, involving only the abstract harmonic designation.
[1.1.3] The way of dealing with counterpoint is a critical morphological choice: theorists who model their systems more closely on linguistic ones, such as Baroni and Jacoboni (1978, 1983), Keiler (1977), and Lerdahl and Jackendoff (1983), have typically chosen non-contrapuntal representations. These include not only monophonic morphologies (e.g., Baroni and Jacoboni; Neumeyer 2009(5)) but also homophonic ones. Although homophonic morphologies like Lerdahl and Jackendoff’s or Kassler’s (1967, 1975) do not necessarily ignore the existence of individual voices and can recognize some kinds of voice leading, they do not separate simultaneous notes as distinct musical events, so that there is a single unified structural description for a passage, which applies equally to all voices. Truly contrapuntal theories that permit the temporal overlapping of distinct musical events include Rahn (1979), Smoliar (1980), Baroni, Dalmonte and Jacoboni (2003), and Brown (2005).
Example 1. Schenker’s analysis of the second movement of Beethoven’s op. 10 no. 3 (1979, Fig. 39/2), showing deep structural disjunctions between the bass and upper voice in mm. 1–7 and mm. 13–21
(click to enlarge)
[1.1.4] When reviewers (such as Hantz 1985, 201) compared Lerdahl and Jackendoff’s (1983) analyses to Schenker’s, they found not only that they differed, but that Schenker’s analyses were not even representable within Lerdahl and Jackendoff’s formalism. The reason is that Schenker often gives differing structural descriptions of different voices,(6) causing them to be realigned at later levels. For instance, in the analysis shown in Example 1 (1979), the principal structural notes in the bass of the exposition occur in mm. 1 and 21. The upper voice has events of a lower structural order in these measures; its principal notes occur in mm. 7 and 13, appearing in less structural chords, especially the applied diminished seventh of m. 7. Schenker realigns these in the middleground level shown on the upper staff, combining notes that come from chords widely separated on the musical surface.
[1.1.5] While a non-contrapuntal approach greatly simplifies the problem of defining and evaluating a generative system, some shortcomings redirect us back towards the more complex morphology required by Schenker: first, that such a theory cannot deal directly with even moderately contrapuntal music suggests a lack of generality.(7) More importantly, however, even in relatively homophonic music, the ability to realign voices at various levels of structure is often what makes it possible for Schenker to find compellingly linear organization beneath many a foreground. Cohn (1985, 45–48) provides an excellent discussion of this issue and additional arguments that cast doubt on the efficacy of non-contrapuntal approaches. The problem of alignment surfaces in some examples from Lerdahl and Jackendoff’s own analyses (1983, 155, 260–3), where they apply their “transformation,” inserting a middleground harmony as an imaginary foreground event, to address misalignments between voices on the foreground (see also Lerdahl 2001, 36–37). Schenker’s own analyses provide numerous instances, such as Example 1 above, where much deeper realignments are essential to demonstrating plausible long-range connections. A dramatic example is his analysis of the “Marcia funebre,” the third movement of Beethoven’s Piano Sonata op. 26 (Schenker [1935] 1979, Figure 40/6): a direct connection from the initial tonic to the dominant at the half cadence (m. 20) is melodically implausible. Schenker solves the problem by realigning the melodic of the cadential viio7/V (mm. 17–19) with the initial tonic, a “fusion” to which the entire extravagantly chromatic progression of mm. 1–16 is subordinate. Such large-scale unfoldings defy any attempt to seal off the horizontal dimension from the vertical.
[1.1.6] The main goal of this article is to address just this problem, which will be tackled directly in parts 3 and 4. As the foregoing discussion has made clear, though, the problem relates to musical hierarchy as well as counterpoint. It is primarily the simplicity of describing music in terms of a single unified hierarchy, directly anchored to literal musical events at all levels, that compels researchers like Lerdahl and Jackendoff to work from a non-contrapuntal morphology. To that end, the next section asks in what sense tonal structure, particularly Schenker’s conception of it, is hierarchical.
2. Tonal Hierarchy
[2.0.1] For Brown (2005) and others such as Rahn (1979) who define tonal structure by means of fully worked-out generative processes, the nature of tonal hierarchy warrants no special mention in the development of the generative framework because it is assumed that the generative process itself confers sufficient hierarchical representation to the analysis. To some extent this is true: one can read implicit hierarchical relationships into some derivational rules, such as the dependency of a neighbor note upon the note that generates it. The implicit hierarchical relationships might not be quite so obvious for other derivations though, particularly those that relate different voices, such as linear progression, unfolding, Übergreifen (“reaching over”) and Untergreifen (“motion from an inner voice”).
[2.0.2] Defining tonal hierarchy is not entirely trivial: the diversity in basic forms of hierarchy that have surfaced in the literature on systematic structural analysis shows that there is little consensus even on its most fundamental features. The best-known formal description of tonal hierarchy comes from Lerdahl and Jackendoff’s Generative Theory of Tonal Music. The basic form of hierarchy described there is also used by many others before and after, such as Komar (1971), Keiler (1977), and Marsden (2005, 2010). Although all of these theories are strongly influenced by Schenker, and might be seen to have had an impact on how Schenkerian analysis is currently taught and understood, none of them are, strictly speaking, formalizations of Schenkerian analysis itself. And indeed, the model of hierarchy upon which they are based is not the one implied by Schenkerian notation. I will make the case here that the latter is more intuitively satisfying as well as more theoretically tractable.
2.1. Representational Hierarchy versus Dynamic Hierarchy
Example 2
(click to enlarge)
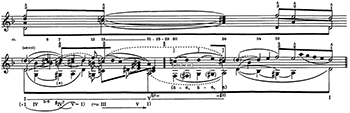
[2.1.1] Cohn and Dempster refer to the kind of model Lerdahl and Jackendoff use as a “representational hierarchy,” meaning that particular tonal events are retained as representatives of sequences of events at higher, or more background, levels (1992, 162). A more complete description would be “representational hierarchy of musical events.” The label “representational hierarchy” suggests that the alternative form of hierarchy might be a non-representational hierarchy with the same kinds of objects (events). But another important alternative is a hierarchy that takes tonal motions, rather than events, as its objects. Following Yust 2006, I will call a hierarchy taking motions as objects a “dynamic hierarchy,” referring to the fact that it organizes the actions of musical events in time hierarchically, rather than events as static objects.(8) These distinctions are best understood with reference to an example. To that end I will give three descriptions of the simple melodic succession in Example 2a using, first, a representational hierarchy of events, then a non-representational hierarchy of events, and finally a dynamic hierarchy (a non-representational hierarchy of motions).
[2.1.2] Example 2a consists of two passing motions, one embedded within the other. A representational model of hierarchy, as in Example 2b, shows this by a reductive process. Each event of the music appears as a leaf (terminal node), and they are successively pruned by retaining an adjacent event at the next-higher level and attaching it to the dependent event. Example 2b shows the embedded passing motion by first pruning the lower-level passing tone, then the higher-level one. Note, however, that the relative vertical position of nodes that are not directly related is irrelevant. The analytic claims of this kind of hierarchy are of the form “Event X is representative of the succession of events Y–X.”
[2.1.3] The non-representational “inclusional hierarchy of events” in Example 2c is the same tree as the representational hierarchy; only the labeling of nodes is different. This difference is not insignificant: in the representational hierarchy an additional choice is made at each level of structure as to which note is more “representative” of the pair, a choice that is not made in the inclusional hierarchy. Also, the inclusional hierarchy misses an important characteristic of most structural theories of tonality, the possibility of parallelism between levels (here, the parallelism of the two passing motions). Nevertheless, the difference is not foundational; it would be more accurate to say that the “representationalism” is an added feature over the basic form of a binary hierarchy of score-objects.
[2.1.4] Example 2d shows 2a as a dynamic hierarchy. This hierarchy is inclusional, not representational, but rather than taking events as its objects it takes motions from one event to another. These correspond directly to slurs or beams in Schenkerian notation. The hierarchy is determined by containment: if one motion contains another temporally, then there is a hierarchical connection from the larger motion to the smaller one.
[2.1.5] Trees, however, are inefficient and not easily readable ways to show dynamic hierarchies. There is a lot of redundancy in a representation like that of Example 2d; the same events often occur as endpoints of multiple motions. The network representation of Example 2e, where events correspond to points (nodes) and motions as lines between them (edges), is more compact and easily readable. A hierarchical connection is implicit wherever edges form a triangle. Events are in temporal order from left to right and more structural motions are always above. This kind of “tree of edges” is called a “two-dimensional tree” or “2-tree” in mathematical graph theory (Harary and Palmer 1973, 73–79), but a more specific term is “maximal outerplanar graph” or “MOP.”(9) It will be the standard notation for this kind of hierarchy throughout this paper.
[2.1.6] The dynamic form of hierarchy is fundamentally different than hierarchies of events; it is impossible to derive one from the other.(10) It is recursive without the added mathematical complexity of representationalism.(11) The representational hierarchy shown in Example 2a claims that the passing eighth-note A “is derived from” or “stands in for” the note G that follows it. Passing motions are asymmetric in both the representational and inclusional hierarchies, as in 2a and 2c. The dynamic hierarchy of Example 2e instead claims that A “moves from B to G” or “delays the arrival at G from B” or “fills in the motion of a third.” In a dynamic hierarchy, the relationship of a passing note to the interval it fills in is symmetric.(12)
Example 3.
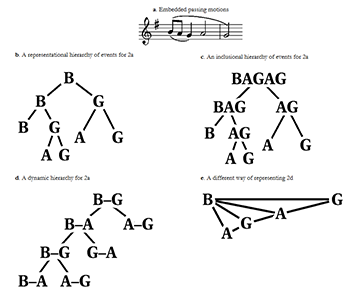
(click to enlarge)
[2.1.7] Dynamic hierarchies are standard for theories of meter. Example 3a reproduces the representational hierarchy of Example 2b as a hierarchy of timepoints: the note durations stand in for timepoints of attack, according to their horizontal position. It would be an unusual theory of meter that required timepoints to be derived from, or stand in for, other timepoints. Instead, the standard approach to modeling meter is a hierarchy of timespans (“motions between timepoints,” so to speak) determined by containment.(13) Example 3b gives such a hierarchy for the rhythm of Example 2a, which is the same tree as the one for pitches in Example 2c. Rather than using noteheads, however, we could use line segments to represent timespans, as in Example 3c. Or, better yet, we can collapse all the points in 3c that represent the same timepoint, as in Example 3d. This is the same MOP as Example 2e.
[2.1.8] It is perhaps the way musical notation reifies the tonal event that makes hierarchies of events seem like candidates for tonal hierarchy, even though they have never been considered plausible metrical hierarchies. The basic score-object, the note, consists of an absolute pitch value and a relative time value, making these both seem more real as musical objects.
2.2. Dynamic Hierarchy and the Grammar of Schenkerian Symbols
Example 4
(click to enlarge)
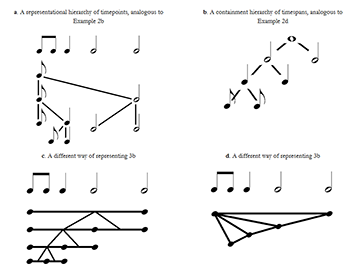
[2.2.1] The symbolic notation of Schenkerian analysis generally follows the logic of dynamic rather than representational hierarchy. Example 4a is part of an analysis that Lerdahl (2001) gives to illustrate prolongational structure. Example 4b is a simple Schenkerian analysis of the same short passage. The Schenkerian analysis shows a fourth-progression in the bass of the first measure. This motion does not appear in Lerdahl’s analysis because a choice must be made as to which tonic chord the dominant in m. 2 elaborates, and Lerdahl chooses the tonic chord at the fermata. Furthermore, the IV6 chord is not a passing chord in this non-existent motion, but a stand-in for the following dominant, while the V6 is a representative of the preceding tonic, so that in the middle of this simple passing motion there is a deep split in the branching structure of Lerdahl’s tree. This means that Lerdahl interprets the two sixth chords that constitute this passing motion to be quite distantly related. Narmour (1977, 96–107) makes a similar point in his critique of Schenkerian tonal hierarchy, which he characterizes as a hierarchy of events. However, since the dynamic model better describes Schenker’s theory, Narmour’s Schenker is a straw man.
[2.2.2] Schenkerian symbols, like slurs and beams, are designed primarily to connect notes rather than to show binary dependencies between them. Consider the soprano of Example 4b. A slur connects F to the C in m. 2, and two shorter slurs connect F to A and A to C. If these slurs were intended to show binary dependencies, this analysis would violate the principle of hierarchy, as shown in Example 4c.(14) (There is no unique path upward from A.) But there is nothing wrong with this use of slurs; they indicate that A fills in an arpeggiation from F to C. Similarly, the function of the slur in the bass connecting F to C is not to indicate a dependency between F and C but to indicate a dependency of the other bass notes (E,
[2.2.3] The implicit syntax of Schenkerian slurs and beams—the distinction between grammatical and ungrammatical usages of them—also reflects the logic of dynamic hierarchy. This close correspondence to Schenkerian notation has made dynamic hierarchy useful for discovering statistical regularities in published Schenkerian analyses (see Kirlin and Jensen 2011, Kirlin 2014, 2016). For instance, Example 4d is ungrammatical because of the crossing slurs, which violate the principle of hierarchy of motions: there is a motion G–A, contained in both A–A and G–
[2.2.4] Example 4e shows the structure implicit in the notation of Example 4b, a hierarchy of motions, each of which appears in the analysis either as a slur or as a direct adjacency (for the “leaves” at the bottom of the hierarchy). We can draw this hierarchy as a tree of slurs, but the “MOP” or 2-tree representation is more compact and visually compelling.
[2.2.5] For Schenker, the dynamic form of tonal hierarchy has even a metaphysical significance, which can be seen in the way his understanding of tonal structure develops within the concept of Urlinie. A milestone document in this development is Schenker’s “Further Considerations of the Urlinie” essays ([1925] 1994, 104–113; [1926] 1996, 1–22), where Schenker formulates the linear progression as the essential component of the Urlinie. In the second essay Schenker summarizes the general idea:
The conceptual unity of a linear progression signifies a conceptual [or psychological] tension between the beginning and the end of the progression: the primary note is to be retained until the point at which the concluding note appears. This tension alone engenders musical coherence. In other words, the linear progression is the sole vehicle of coherence, of synthesis. ([1926] 1996, 1; Schenker’s italics)
As Korsyn (1988) has shown, Schenker’s use of the terms “coherence” (Zussammenhang) and “synthesis” (Synthese) references Kant, who derives consciousness from cause and effect as the temporal unification otherwise independent acts of perception. In Schenker’s musical formulation, the teleology of the passing-tone formation binds musical timespans into a coherent unity analogous to consciousness itself. The “retention of the primary tone” is essential to this synthesis, which is represented by the slur (or beam), showing that all of the musical content is subsumed by an ongoing process that guarantees the coherence of the whole.(16) The temporality of the objects of dynamic hierarchy is therefore crucial to the philosophical basis of Schenker’s theory.
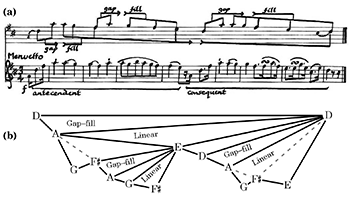
Example 5. Leonard Meyer’s (1973, 103) analysis of the Menuetto from Mozart’s Flute Quartet, K. 298 (a), and a network representation of the hierarchy (b)
(click to enlarge)
[2.2.6] Nonetheless, dynamic hierarchy is not exclusive to Schenker’s theory or its metaphysical concerns. Leonard Meyer’s implication–realization hierarchies, for example, are also dynamic. Example 5 reproduces one of Meyer’s (1973) illustrations that shows how multiple melodic gap–fill processes can embed one another. Meyer’s beams, an adaptation of a Schenkerian notation, reflect a dynamic connectivity to his concept of process.(17)
[2.2.7] The simplicity of dynamic hierarchy, relative to the representative hierarchy, is also an advantage for the construction of generative systems, a point made by Mavromatis and Brown (2004) and Gilbert and Conklin (2007). A representational model multiplies the number of possible structures for a given sequence of events by a substantial factor, making additional musical distinctions that are often not musically significant for basic diminution-type operations like passing or neighbor notes. For instance, one usually considers the neighbor note to be a unitary phenomenon, but under a representational model of hierarchy there are two distinct neighbor-note operations, left-branching and right-branching. The added complexity of the representational model leads to a large number of possible analyses, as noted by Marsden (2005, 2010), and at least partly accounts for the excessive number of satisfactory structural descriptions found by Marsden (2010) in algorithmic applications of Schenkerian analysis using representational hierarchies.
3. Voice-Leading Transformations
[3.0.1] In part 1, I argued that the status of counterpoint within a stratified conception of tonality is a particularly pressing matter for theories of tonal structure. The distinction between vertical and horizontal is clearly essential to Schenker’s theory, and yet they cannot be neatly isolated from one another in his derivational processes. Proposals for more forthrightly systematic approaches to tonal structure have often avoided the more radically contrapuntal features of Schenker’s theory, and for good reason: coordinating voices in a derivational process so as to prevent tonal relationships from being undermined at subsequent levels is a delicate problem.
[3.0.2] This section tackles this problem by first considering some insights of Schenker’s that advocate for the strongly contrapuntal flavor of his analytical approach. We will then see how a keystone of his late theory, the principle of Stimmführungsverwandlungen (voice-leading transformation), serves as a way of handling the interactions between harmony and contrapuntal voice. The role of contrapuntal voices in the distinction between transformation and diminution in Schenker’s derivations provides insight into his late analytical practice and its historical development. By defining a precise procedure of voice-leading transformation using the dynamic model of hierarchy described above, we can sketch a theory of tonal structure that incorporates some of the most elusive yet musically compelling aspects of Schenker’s approach. A more thoroughgoing analysis presented in part 4 demonstrates the value of such theoretical rigor.
3.1. The History of Schenker’s Stimmführungsverwandlungen
[3.1.1] Schenker’s concept of voice-leading transformation, developed while he was working on Free Composition in the later 1920s, is foundational to the theory for which he is now known. While the method of reducing musical surfaces is not unique to Schenker, and the term Urlinie has a deeper history reaching back to Schenker’s critical edition of Beethoven’s Piano Sonata op. 101 (1970), first published in 1921, the term Ursatz appears first in Schenker’s commentary on his analysis of J.S. Bach’s Little Prelude no. 5 in Tonwille V (Schenker [1923–4] 2005, 180–1, 212–13; see also Pastille 1990). In Schenker’s Tonwille essays, the meaning of his term Urlinie was different than in his later theory (and current usage); it refers to an underlying stepwise line, but not necessarily one that was uniformly descending or succinct. Indeed, these earlier Urlinien could meander at some length (see Clark 2009; Lubben 1995, 65–86; Pastille 1990). In his earliest published exegesis of the concept, he compares the repeated ups and downs of the Urlinie to inhaling and exhaling. (Schenker [1921] 1970, 8)
[3.1.2] The idea Schenker hit upon in his analysis of Bach’s modest Little Preludes that led to the concept of Ursatz and the theory of Free Composition was what he calls Stimmführungsprolongationen (voice-leading prolongations) in those essays. This technique involves a vertical arrangement of musical lines and counterpoints beginning with a simple counterpoint on top and gradually deriving more musical content proceeding downward, until arriving at something recognizably close to the musical surface of the piece. While the analyses of Little Preludes nos. 3 and 5 (Schenker [1921–3] 2004, 175–6, 180–1) are the first applications of this technique to entire pieces, more limited instances appear in earlier essays.(18) The method is used specifically to reveal underlying contrapuntal structures, and is connected with the theory of prolongation developed in the second volume of Counterpoint (1987).(19) Schenker’s earlier focus on Fuxian teaching and contrapuntal rules in the Counterpoint volumes did not yield a punchy argument against a theory of chord successions. The Stimmführungsprolongationen achieved this by invoking counterpoint in a more abstract sense, allowing Schenker to assert the primacy of linear organization by reconceptualizing contrapuntal voices as contingent upon hierarchical level. From this point onward, these types of graphs became a staple of his analytic technique (see Yust 2006, 10–19, 31–39).
[3.1.3] Schenker’s development of this theory through the analytical use of these voice-leading prolongation graphs gradually led to the idea that became the basis of his theory of levels and the core principle of his late theory, that of voice-leading transformation. Voice-leading transformation, or “horizontalization,” refers to the linearization of vertical intervals, in which multiple voices at one level collapse to a single voice at a subsequent level. It implies that contrapuntal voices only exist relative to their structural level. The redefinition of contrapuntal voices from one level to the next through voice-leading transformations allowed Schenker to extend his accounts of tonal structure to the ultimate background of a musical work while describing each individual level in purely contrapuntal terms.
[3.1.4] This principle, while central to Schenker’s own theory, is not a requirement for structural theory more generally. Some approaches to tonal structure (Keiler 1977, Lerdahl and Jackendoff 1983) ignore it entirely, instead developing a structural theory of chords. Others (Brown 2005, Rahn 1979) give a primary role to linear relationships without invoking the larger-scale implications of a more robust notion of contrapuntal voice. It is tempting to assume that robust notions of hierarchy and voice automatically fall out from the generative and linear aspects, respectively, of these latter approaches. This is not so, however, and one significant reason is that we must address the tricky question of how hierarchy and voice interact.
3.2. Contrapuntal Hierarchy
Example 6
(click to enlarge)
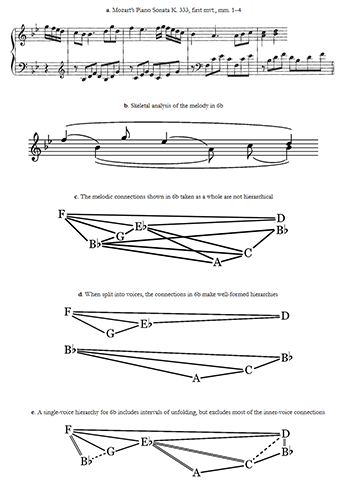
[3.2.1] Voice-leading transformations may be seen as a generalization of principles of compound melody. The melody of the first movement of Mozart’s Piano Sonata K. 333 in Example 6a, for example, outlines the counterpoint of two voices shown in Example 6b.(20) If one treats the melody as an undifferentiated whole, the melodic connections in the analysis violate the principle of crossing slurs and do not amount to a well-formed hierarchy, as shown in Example 6c. The melodic connections are hierarchical only when separated into distinct voices, as in Example 6d.
[3.2.2] Yet the separation into voices forces us to abandon some connections shown in the analysis—the unfoldings F–
[3.2.3] Such examples of compound melody at the foreground make a strong case that at least some interaction between counterpoint and hierarchy is necessary for a musically satisfying account of tonal structure. For Schenker, however, the important forms of voice-leading transformation are those that occur in derivations of deeper middleground structures. His middleground techniques of unfolding, linear progression, reaching over, motion from an inner voice, and initial ascent all refer to forms of voice-leading transformation.(21)
[3.2.4] Voice-leading transformations have profound implications for the formal characteristics of tonal hierarchy. The simple models of hierarchy outlined in part 2, while serving as a good starting point for the investigation of how tonal hierarchy works, are not sufficient by themselves to fit Schenker’s understanding of tonal structure. The situation is analogous to the transformational grammars of linguistics. Chomsky (1957) famously demonstrated that while simple hierarchies (generative grammars) explain a great deal of how language works, certain features of language can only be explained by well-defined operations (transformations) applied to the hierarchies created by generative grammars. Similarly, the phenomenon of compound melody shows that some tonal formations do not conform to the model of simple hierarchy. Relationships of a simple hierarchical character (diminutions like passing and neighbor tones) can be derived by a generative grammar while others involve well-defined transformations on the simple hierarchies created by the generative grammar.
Example 7
(click to enlarge)
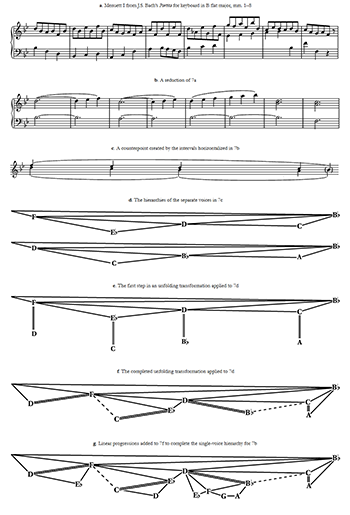
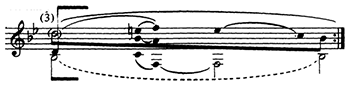
[3.2.5] The analysis in Example 7 breaks a voice-leading transformation into stages to illustrate the process of combining contrapuntal lines. Example 7a is a phrase from a Bach Menuett from the Partita in
[3.2.6] The voices in the simple counterpoint of Example 7c have the same structural outline, as shown in Example 7d. Note that a placeholder vertex has been added to this structure before the first note. Such placeholder vertices can be put in the upper left-hand and/or upper right-hand corner to denote structural beginning and structural ending (see Yust 2006, 40–44). An unfolding transformation can be applied when the two voices share a background edge at the top, which could involve one or two placeholder vertices. Here the shared edge is from a placeholder vertex to the common note
[3.2.7] The unfolding transformation begins by eliminating all of the edges of the non-principal voice and conflating the two vertices on the uppermost edge of each voice. The new structure begins with the principal voice’s structure on top (here, • –F–
3.3. Schenker’s Structural Levels
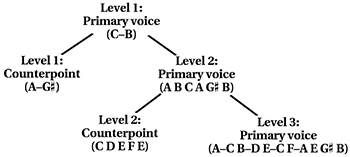
[3.3.1] The principle of voice-leading transformations has deep consequences for the concept of voice. Voices exist not only at the foreground but also at deeper levels, with each level having its own distinct set of voices. Schenker himself seems to have been aware of this logical consequence of his ideas of unfolding and linear progression. In Free Composition, Schenker devotes a section (§48) to “The number of structural levels,” stating that “in each individual instance the number [of structural levels] can be specified exactly” (26). Such an assertion makes no sense under a simple-hierarchy view of tonal structure (or what Proctor and Riggins (1988) call “severe reductionism”). Under a simple hierarchy, where one draws the line between distinct “levels” is largely arbitrary, making the idea of counting levels senseless. For this reason, Schachter (1981, 120–21) expresses befuddlement at this paragraph of Free Composition. The levels that Schenker has in mind, however, are specifically those created by voice-leading transformations, not by the many generative rules, or “diminutions,” such as passing and neighboring motion. While an analysis might involve a multitude of diminutions, the number of voice-leading transformations is limited, since each one defines a new set of contrapuntal voices at a subsequent level.
[3.3.2] Schenker also says in §48 that his own analyses substantiate his claim about the number of structural levels, but cites no specific example. The analyses he published from 1926 onward as a group bear out the idea that he regarded voice-leading transformations as the basic distinguishing feature between structural levels. Three of the Five Graphic Music Analyses (Schenker [1932] 1969) derive middlegrounds through a series of three levels (Bach “Ich bin’s, ich sollte büssen” and the Chopin Etudes op. 10 no. 8 and no. 12), and in each case Schenker indicates prominent unfoldings or linear progressions to explain the relationships between levels. The first analysis (of the Bach chorale) is especially illustrative, because Schenker gives distinct visual emphasis to the embedded unfoldings in his graphs. The analysis of the first movement of Beethoven’s “Eroica” Symphony in Schenker ([1930] 1997, 10–68) is similar in that middleground graphs are explicitly labeled “first level” (“erste Schicht”), “second level,” etc., and derived through prominent voice-leading transformations, including linear progressions, reachings over, unfoldings, and initial ascents. In the graphs of the first movement (10–11), for example, Schenker marks prominent descending linear progressions in the upper voice added at level 2, and adds unfoldings in the bass at level 3.(22) Similar observations can be made in other extended analyses from Meisterwerk II (Schenker [1925] 1994, 56, 60–61, 80–81, 91, 94–95), where linear progressions are usually the prominent level-distinguishing features.(23)
[3.3.3] We can differentiate Schenker’s analyses based on voice-leading transformation (which he specifically refers to as “Stimmführungsverwandlungen” or “Stimmführungs-schichten”) from earlier voice-leading prolongation graphs, such as the analyses of Bach’s Little Preludes. Voice-leading prolongations, relating to the ideas in Counterpoint II (Schenker [1922] 1987) involve a wider range of more specific operations that change species or introduce an element of free composition such as chromaticism.(24) Schenker’s transition from Stimmführungsprolongationen to Stimmführungsverwandlungen fits the trajectory described by Lubben (1993, 1995) whereby Schenker’s motivation for reductive thinking in Tonwille—demonstrating the laws of strict counterpoint underlying free composition—gives way as this reductive thinking develops into the more systematic theory of Der freie Satz. The distinction between prolongation and transformation emerges gradually as Schenker refines the Stimmführungsprolongationen method in the analyses of Tonwille. For instance, in his analysis of the recitative “Erbarm es Gott” from Bach’s St. Matthew Passion (Schenker [1923–4] 2005, 65–66) he refers to reaching over as a “transformation,” and in his analysis of the theme of Brahms’s Variations and Fugue on a Theme of Handel, op. 24 (Schenker [1923–4] 2005, 77–78), he explicitly refers to a “first level” and “next level” in voice-leading prolongational analysis that consists of an Urlinie plus six stages of elaboration. The point where the division between levels occurs (from his Figures 1c to 1d) is explicitly shown as a voice-leading transformation, where four voices are collapsed into two by horizontalizations in the upper voice.(25)
[3.3.4] This development towards a theory of voice-leading transformation can be traced in the changing meaning of Schenker’s terms for linear progressions: Quartzug, Quintzug, etc. In analyses from the first two volumes of Der Tonwille Schenker uses the terms for motivic elements of the Urlinie that do not necessarily involve the composing-out of a harmony.(26) In the later theory, the element of horizontalization, the composing-out of an interval of harmonic significance, is essential to the Zug concept.(27) The idea of horizontalization emerges fairly early within this historical window. It can be identified as early as Tonwille IV–V in the analyses of Bach’s Little Preludes nos. 1, 3, and 5 (Schenker [1921–3] 2004, 141, 175–6, 180–1), in the same set of essays as the first thoroughgoing use of the Stimmführungsprolongationen technique (see [3.1] above). However, the concept of horizontalization was not united with that of Zug until somewhat later, first appearing in two of the analyses in Tonwille 10,(28) and being consolidated into a theory of voice-leading transformation in the “Further Considerations of the Urlinie” essays.(29) Even in Tonwille 7 Schenker refers to a “third-progression” (“Terzzug”) in Beethoven’s Piano Sonata op. 57 that is motivic but outlines an interval between dissonant notes (Schenker [1923–4] 2005, 44).(30) While the difference between “passing motions” filling in horizontalized “tonal spaces” (e.g., Schenker [1921–3] 2004, 175; 2005, 77–78, 117–18) and linear progression might seem at first like a minor semantic difference, the frequent use of linear progressions to describe directed elements of Urlinien in many of Schenker’s analytical essays up to that point made it an epiphany, because it disrupts the fixity of the Urlinie/inner-voice distinction that his analytical method, and concepts of reaching over and motion to an inner voice, had presumed up to that point.(31)
3.4. An Example of Multiple Voice-Leading Levels
Example 8
(click to enlarge)
Example 9. The hierarchy of voices created by the transformations in Example 8
(click to enlarge)
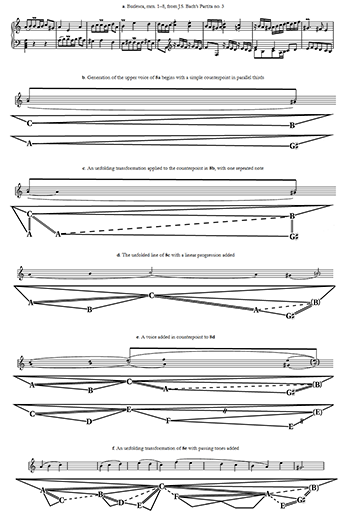
[3.4.1] The melodic analysis of J.S. Bach’s Burlesca from the A-minor Partita (Clavier-Übung I, no. 3, BWV 827) in Example 8a illustrates how, through a series of voice-leading transformations, a melody can be stratified into multiple levels. At the deepest level, the melody is based on a simple interrupted line C–B, and a contrapuntal line in parallel thirds, A–
[3.4.2] Use of voice-leading transformations implies that a simple tonal hierarchy by itself is not an adequate description of tonal structure. Instead, there are multiple simple hierarchies, one for each voice at each level, and the voices themselves participate in a single, more abstract, hierarchy of voices, a “hierarchy of hierarchies,” so to speak. The operations that generate content within the simple hierarchies of a given level are the various simple diminutions like passing and neighbor notes, repeated notes and arpeggiations. Voice-leading transformations operate between levels, and, by deriving voices at one level from the previous one, form a hierarchy of voices like the one in Example 9, for the three levels of structure outlined in Examples 8b–f for Bach’s Burlesca. This kind of hierarchy is fundamentally different from the simple tonal one: it exists out of time, so that left and right branchings do not indicate temporal precedence. Rather, the left branchings indicate new voices generated in counterpoint to previous ones at the same level, while right branchings are reserved for voice-leading transformations and create a new level of structure. The voices group along the diagonals to form individual levels.
4. A Structural Dissonance in Beethoven’s Op. 22 Menuetto
[4.0.1] This part implements the theory of counterpoint developed above by working through a short analysis in detail. In addition to illustrating the use of voice-leading transformations, this analysis demonstrates how well-defined theoretical premises clarify the implications of analytical decisions and lead to observations not accessible from a more naïve perspective. After gaining a familiarity with such derivations, one need not necessarily work out every one with such exacting detail to recognize the significant analytical questions posed by the underlying theory.
4.1. Finding the Structural Counterpoint
Example 10. mm. 1–8 of the Menuetto from Beethoven’s Piano Sonata op. 22, third movement
(click to enlarge)
Example 11. Schenker’s analysis of mm. 1–8 (Free Composition, fig. 82/2)
(click to enlarge)
Example 12. A reduction of the Menuetto with two possible choices of structural inner voice (a), and some structural consequences of each choice (b–c)
(click to enlarge)
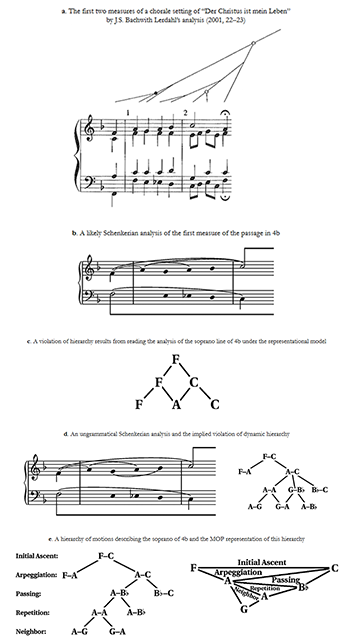
[4.1.1] The excerpt from the third movement of Beethoven’s Piano Sonata op. 22 in Example 10 is a short eight-measure period that, as the first part of a sectional rounded binary, expresses a complete structure. These eight measures return with only minor variations as mm. 17–24, bringing the piece to structural close. This being Beethoven, even this relatively simple Menuetto pushes the boundaries of tonal practice: the insistent G at the high point of the melodic line lingers over the second phrase as an unresolved ninth. The analytical investigation below suggests that this is in fact a deeply structural feature.
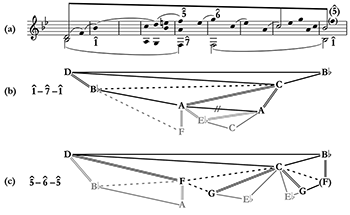
[4.1.2] Schenker’s analysis of this theme from Free Composition (Example 11) is only a rough outline. However, one notable feature is his exclusion of the prominent ninth (G) in the extended dominant of the second phrase, which implies that he interprets it as a relatively foreground phenomenon.(32) While it is reasonable to guess that such an unstable dissonance would not attain a very deep level of structural significance, that assumption turns out to be contrary to the most distinctive features of Beethoven’s melody. Most obviously, the G is clearly present over the dominant throughout mm. 5–7, appearing in m. 5 and m. 7 with no resolution to F implied anywhere in those measures. The reemergence of G just before the cadence challenges Schenker’s reading, since it cannot be in the same voice as the
[4.1.3] To focus attention on what is at stake in the analysis, Example 12 provides a preliminary reduction and sketch. The location of Urlinie elements, shown in the sketch, is unambiguous given the limited number of possibilities offered by the simple harmony (particularly the fact the entire second phrase up to the cadence unfolds over a single dominant chord), and agrees with Schenker’s Urlinie. The reduction makes it apparent that the theme consists essentially of melodic motion within the tonic in the first phrase and motion within the dominant in the second phrase. Working from the theory of levels explained above, our first question should then be: Which of these melodic motions represent the unfoldings that define the first middleground level? Since these unfoldings will relate two coherent lines, we are in essence asking, What is the structural counterpoint to the Urlinie?
[4.1.4] There are two possible structural inner lines in Example 12a: the typical one for a 3-line, a –– neighbor motion, can be found from the
[4.1.5] Example 12(b–c) shows the immediate consequences of each choice after unfolding the first counterpoint and some elements of a subsequent level. The conventional structural counterpoint, ––, implies that the first structural unfolding occurs within the first two measures, from D to
[4.1.6] The analysis that ensues from the choice of the –– structural counterpoint in Example 12c is, in contrast to the –– analysis, much more true to the shape of Beethoven’s melody. The first unfolding (D–F) spans the entire unified ascending gesture of the first phrase. The next unfolding (G–C) outlines the sequential melody that dominates the second phrase. Even the second ascent to the G in m. 7 now has a neater structural description as the second unfolding of the C–G interval.
[4.1.7] The purpose of such deliberation is not primarily to make us feel comfortable asserting that this piece displays an unusual structural feature (the structural –– counterpoint), one that, as we will see below, has significant motivic consequences for the rest of the Menuetto. Rather, it demonstrates what features Beethoven may have brought to bear to substantiate such an interpretation. If, indeed, this analysis is accurate, then the evidence supporting it also points to the exceptional features of the music that constitute the content of this claim. These include the unity of melodic gesture approaching the high F in the first phrase, the shape of the sequence descending from the dissonant G in the second phrase, and the reappearance of the G just before the cadence in the second phrase.
4.2. Echoes of the Structural ––
[4.2.1] Another striking feature of the Menuetto theme that the analysis in Example 12c highlights is the lack of a literal resolution for the dominant’s dissonant ninth, G. This is shown by the necessity of an implied F to complete the structural inner voice. Since this implied resolution occurs at a later level than the Urlinie, it does not mean that cadential resolution is withheld. However, it does entail an extra-structural tension, a loose end left behind, with significant ramifications in the second part of the piece. The tonal activity of the contrasting middle and coda are largely motivated by a desire for a more satisfying resolution of the structural inner voice.
Example 13
(click to enlarge)
Example 14. G–F resolutions in the coda, mm. 24–30
(click to enlarge)
[4.2.2] In the contrasting middle, mm. 9–16, Beethoven repeats the G–F motion, as summarized in Example 13. Here the G pulls more tonal weight by having the three tonicized areas (G minor,
[4.2.3] The implied resolution and superposition of the inner voice once again leave a residue of extra-structural tonal tension in m. 16 that remains after the recapitulation of the main theme in mm. 17–24. The imperative is then upon the coda (mm. 25–30, Example 14) to lay this extra-structural tension to rest. It does so by featuring a series of prominent G–F resolutions in an imitative texture. Beethoven ingeniously deploys the imitative texture as a way to transfer the G–F resolution into an obligatory register below the Urlinie.
[4.2.4] One might be tempted to call this a motivic –,(33) but according to the structural analysis it is a more specific process. The multiple appearances of the – resolution (as the structural inner voice of mm. 1–8, mm. 9–16, and mm. 17–24, and finally in the coda) are not simply repetitions but a teleologically directed tonal process working out the instability created by the use of register and implied resolution.
4.3. A Complete Derivation
[4.3.1] This section presents a full derivation of the Menuetto theme to demonstrate the efficacy of a generative process and the soundness of the analysis outlined in Example 12c. In general, such painstaking detail is not always necessary in using the theory presented in part 3 to draw the kind of analytical conclusions described in the first section of part 4. I include it here as proof of concept and a further demonstration of voice-leading transformations and hierarchies of voices.
Example 15
(click to enlarge and see the rest)
Example 16. The hierarchy of voices involved in the transformations from 15b to 15f
(click to enlarge)
[4.3.2] The graphs of Example 15a work out the specifics of the analysis in Example 12c. Levels 0–1 derive the Ursatz as the unfolding of the “chord of nature” (Schenker [1925] 1994, 104, 118), while levels 1–2(34) add the superposed inner voice F–G–F and unfold the counterpoint of the two upper voices.(35) This example also introduces a new notation, an “8” drawn over the network edge to show an octave change. At level 2 a basic contrapuntal tension arises in the delay of the structural C over the structural dominant F in the lower voice, which can be seen in the divergent shapes of the two networks. This contrapuntal tension eventually expands to occupy all of mm. 5–7, the span of the dominant ninth chord.
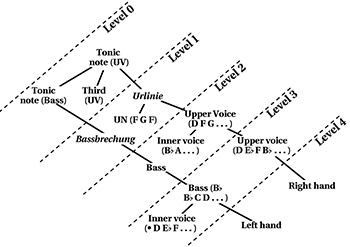
[4.3.3] At level 2 in Example 15b the arpeggiation G–
[4.3.4] The new voice added at level 3 unfolds into the left-hand part at level 4, making level 4, shown in Example 15d, the last one. Though the foreground consists of two voices, traces of earlier levels remain where Beethoven leaves certain voices “folded” near the cadence points while the rest of the counterpoint undergoes its transformation. (This tends to occur near cadence points in Schenker’s analyses too; see, for example, his analysis of the Bach chorale “Ich bin’s, Ich sollte büssen” in Schenker [1932] 1969, 32–3.) The remainder of the derivation is generative, through the addition of diminutions, the most notable of which are shown in Example 15e: the ascending-fifth progressions that end each phrase and the arpeggiations filling in the left hand’s unfolded dissonances in the second phrase.
[4.3.5] A number of distinct voices arise in the course of this derivation through five levels, which can be organized into a hierarchy of voices (Example 16). The voices at level 0 (in the “chord of nature”) are motionless individual notes from the tonic chord. The fact that the upper-voice tonic note generates two voices in counterpoint—trivial counterpoint in this case—before joining with one of them in unfolding means that it leaves one voice (the bass) behind for further independent elaboration. This basic two-part division of voices remains in place through all later levels down to the left-hand/right-hand division of the compositional surface. Level 1 introduces motion through the voice-leading transformation that creates the Urlinie. The topical upper-neighbor motion (F–G–F) also belongs to level 1. An unfolding transformation on the Urlinie and the upper-neighbor line lead to level 2, where the more standard inner-voice counterpoint to the principal line (––) is also introduced. Another unfolding in the upper voice leads to level 3. Through all three of these transformations, the bass voice tags along, adding diminutions but participating in no transformations of its own. This changes at level 3, where the final introduction of a new voice and subsequent voice-leading transformation belongs to the bass. Level 4 represents the two-voice counterpoint of the compositional surface.
5. Conclusions
[5.1.1] Interest in generative theories of tonal structure reached an apex of activity within the music theory community in the 1970s and 1980s, an era whose intellectual climate—especially its confidence in the power of formalism—now seems distant. If the positivism of this earlier era was sometimes overexuberant, it was never entirely misplaced. Theoretical insights often remain inaccessible until the premises of a theory and the meaning of its operations are made explicit and surveyable. The efforts towards systematization carried out here, for example, have revealed a crucial feature of Schenker’s theory that—surprisingly, given the size of the secondary literature—has gone essentially unnoticed: the dependence of his concept of structural level on voice-leading transformation. We have also seen how conceptual rigor benefits the process of interpretation, because it leads us to ask, and gives us the means to answer, questions that likely would not have occurred to the analyst working under a regime of ambiguous and promiscuous concepts. The analysis of Beethoven’s op. 22 Menuetto poses such a question about the structural order of voices underlying a single melody, and the answer not only reveals a unique feature of pervasive motivic significance, but helps us draw a line from aspects of the musical surface to this structural feature, leading ultimately to a deep appreciation of Beethoven’s artistry. Even these points are secondary to what is perhaps the chief benefit of formalization, the possibility of powerful abstractions and generalizations, which we have yet to begin to explore.
Jason Yust
College of Fine Arts
Boston University
855 Commonwealth Avenue
Boston, MA 02215
jyust@bu.edu
Works Cited
Babbitt, Milton. 1965. “The Structure and Function of Music Theory.” College Music Symposium 5: 49–60.
Baroni, Mario, Rossana Dalmonte, and Carlo Jacoboni. 2003. A Computer-Aided Inquiry on Music Communication: The Rules of Music. Edwin Mellen Press.
Baroni, Mario and Carlo Jacoboni. 1978. Proposal for a Grammar of Melody: The Bach Chorales. Les Presse de l’Université de Montréal.
—————. 1983. “Computer Generation of Melodies: Further Proposals.” Computers and the Humanities 17 (1): 1–18.
Brown, Matthew. 2005. Explaining Tonality: Schenkerian Theory and Beyond. University of Rochester Press.
Chomsky, Noam. 1957. Syntactic Structures. Mouton.
Clark, Suzannah. 2009. “The Politics of the Urlinie in Schenker’s Der Tonwille and Der freie Satz.” Journal of the Royal Musical Association 132 (1): 141–64.
Cohn, Richard. 1985. Review of A Generative Theory of Tonal Music by Fred Lerdahl and Ray Jackendoff. In Theory Only 8 (6): 27–52.
Cohn, Richard and Douglas Dempster. 1992. “Hierarchical Unity, Plural Unities: Towards a Reconciliation.” In Disciplining Music: Musicology and its Canons, ed. Katherine Bergeron and Philip V. Bohlman, 156–81. University of Chicago Press.
Cook, Nicholas. 2007. The Schenker Project: Culture, Race, and Music Theory in Fin-de-Siècle Vienna. Oxford University Press.
Dibben, Nicola. 1994. “The Cognitive Reality of Hierarchic Structure in Tonal and Atonal music.” Music Perception 12 (1): 1–25.
Dubiel, Joseph. 1990. “When You are a Beethoven: Kinds of Rules in Schenker’s ‘Counterpoint.’” Journal of Music Theory 34 (2): 291–340.
Farbood, Morwaread M. 2012. “A Parametric, Temporal Model of Musical Tension.” Music Perception 29 (4): 387–428.
Gilbert, Édouard and Darrell Conklin. 2007. “A Probabilistic Context-Free Grammar for Melodic Reduction.” In Proceedings of the International Workshop on Artificial Intelligence and Music, 20th International Joint Conference on Artificial Intelligence, Hyderabad, India, 83–94.
Hamanaka, Masatoshi, Keiji Hirata, and Satoshi Tojo. 2013. “Toward Developing a Polyphonic Music Time-Span Tree Analyzer.” In Mathematics and Computation in Music: 4th International Conference, MCM 2013, ed. Jason Yust, Jonathan Wild, and John Ashley Burgoyne, xiv. Springer.
Hantz, Edwin. 1985. Review of A Generative Theory of Tonal Music by Fred Lerdahl and Ray Jackendoff. Music Theory Spectrum 7: 190–202.
Harary, Frank and Edgar Palmer. 1973. Graphical Enumeration. Academic Press.
Kassler, Michael. 1967. “A Trinity of Essays.” PhD diss., Princeton University.
—————. 1975. Proving Musical Theorems: The Middleground of Heinrich Schenker’s Theory of Tonality. Basser Department of Computer Science, University of Sydney.
Katz, Adele. 1945. Challenge to Musical Tradition: A New Concept of Tonality. Alfred A. Knopf.
Keiler, Allan. 1977. “The Syntax of Prolongation.” In Theory Only 3 (5): 3–27.
Kirlin, Phillip B. 2014. “A Probabilistic Model of Hierarchical Music Analysis.” PhD diss., University of Massachusetts–Amherst.
Kirlin, Phillip B. and Darrell D. Jensen. 2011. “Probabilistic Modeling of Hierarchical Music Analysis.” In Proceedings of the International Society for Music Information Retrieval, Miami.
Kirlin, Phillip B. and Paul E. Utgoff. 2008. “A Framework for Automated Schenkerian Analysis.” In Proceedings of the International Society for Music Information Retrieval, Philadelphia.
Kirlin, Phillip B. and Jason Yust. 2016. “Analysis of Analysis: Using Machine Learning to Evaluate the Importance of Music Parameters for Schenkerian Analysis.” Journal of Mathematics and Music 10 (2). Forthcoming.
Komar, Arthur. 1971. Theory of Suspensions: A Study of Metrical and Pitch Relations in Tonal Music. Princeton University Press.
Korsyn, Kevin. 1988. “Schenker and Kantian Epistemology.” Theoria 3: 1–58.
Larson, Steve. 1997. “The Problem of Prolongation in ‘Tonal’ Music: Terminology, Perception, and Expressive Meaning.” Journal of Music Theory 41 (1): 101–36.
—————. 2004. “Musical Forces and Melodic Expectations: Comparing Computer Models and Experimental Results.” Music Perception 21 (4): 457–98.
Lerdahl, Fred. 1997. “Issues in Prolongational Theory.” Journal of Music Theory 41 (1): 141–55.
—————. 2001. Tonal Pitch Space. Oxford University Press.
Lerdahl, Fred and Ray Jackendoff. 1977. “Towards a Formal Theory of Tonal Music.” Journal of Music Theory 21 (1): 111–171.
—————. 1983. A Generative Theory of Tonal Music. MIT Press.
—————. 1993. “Hierarchical Structure in Music.” In Machine Models of Music, ed. Stephan M. Schwanauer and David A. Levitt, 289–312. MIT Press.
Longuet-Higgins, H. Christopher, and Christopher S. Lee. 1984. “The Rhythmic Interpretation of Monophonic Music.” Music Perception 1(4): 424–41.
Lubben, Joseph. 1993. “Schenker the Progressive: Analytical Practice in Der Tonwille.” Music Theory Spectrum 15, 59–75.
—————. 1995. “Analytic Practice and Ideology in Heinrich Schenker’s Der Tonwille and Cantata Harmonia Mundi.” PhD diss., Brandeis University.
Marsden, Alan. 2005. “Generative Structural Representation of Tonal Music.” Journal of New Music Research 34 (4): 409–428.
—————. 2010. “Schenkerian Analysis by Computer: A Proof of Concept.” Journal of New Music Research 39 (3): 269–289.
Mavromatis, Panayotis, and Matthew Brown. 2004. “Parsing Context-Free Grammars for Music: A Computational Model of Schenkerian Analysis.” In Proceedings of the 8th International Conference for Music Perception and Cognition, Evanston, Ill.: 414–15.
Meyer, Leonard B. 1973. Explaining Music: Essays and Explorations. University of California Press.
Narmour, Eugene. 1977. Beyond Schenkerism: The Need for Alternatives in Music Analysis. University of Chicago Press.
Neumeyer, David. 1987. “The Ascending Urlinie.” Journal of Music Theory 31 (2): 275–303.
—————. 2009. “Thematic Reading, Proto-Backgrounds, and Registral Transformations.” Music Theory Spectrum 31 (2): 284–324.
—————. 1990. “The Development of the Ursatz in Schenker’s Published Works.” In Trends in Schenkerian Research, ed. Allen Cadwallader, 71–85. Schirmer Books.
Pastille, William. 1985. “Ursatz: The Musical Philosophy of Heinrich Schenker.” PhD diss., Cornell University.
Peel, John, and Wayne Slawson. 1984. Review of A Generative Theory of Tonal Music by Fred Lerdahl and Ray Jackendoff. Journal of Music Theory 28 (2): 271–94.
Proctor, Gregory, and Herbert Lee Riggins. 1988. “Levels and the Reordering of Chapters in Schenker’s Free Composition.” Music Theory Spectrum 10, 102–126.
Rahn, John. 1979. “Logic, Set Theory, Music Theory.” College Music Symposium 19 (1): 114–27.
Rothstein, William. 1989. Phrase Rhythm in Tonal Music. Schirmer.
—————. 1990. “The Americanization of Heinrich Schenker.” In Schenker Studies 1, ed. Hedi Siegel, 193–203. Cambridge University Press.
Salzer, Felix. 1952. Structural Hearing. Dover.
Schachter, Carl. 1980. “Rhythm and Linear Analysis: Durational Reduction.” In Music Forum 5, ed. William Mitchell and Felix Salzer. Columbia University Press. Reprinted in Unfoldings: Essays in Schenkerian Theory and Analysis, ed. Joseph Straus, 54–78. Oxford University Press.
—————. 1981. “A Commentary on Schenker’s Free Composition.” Journal of Music Theory 25 (1): 115–142.
Schenker, Heinrich. [1921–3] 2004. Der Tonwille: Pamphlets in Witness of the Immutable Laws of Music, Volume I: Issues 1–5, ed. William Drabkin. Oxford University Press.
—————. [1921] 1970. Erläuterungsausgabe: Die Letzten Sonaten Beethovens, Sonate A Dur Op. 101. 2nd ed., ed. Oswald Jonas. Universal Edition.
—————. [1922] 1987. Counterpoint II, trans. John Rothgeb and Jürgen Thym. Schirmer Books.
—————. [1923–4] 2005. Der Tonwille: Pamphlets in Witness of the Immutable Laws of Music, Volume II: Issues 6–10, ed. William Drabkin. Oxford University Press.
—————. [1925] 1994. The Masterwork in Music: A Yearbook, vol. I, ed. William Drabkin. Cambridge University Press.
—————. [1926] 1996. The Masterwork in Music: A Yearbook, vol. II, ed. William Drabkin. Cambridge University Press.
—————. [1930] 1997. The Masterwork in Music: A Yearbook, vol. III, ed. William Drabkin. Cambridge University Press.
—————. [1932] 1969. Five Graphic Music Analyses, ed. F. Salzer. Dover.
—————. [1935] 1979. Free Composition: Volume III of New Musical Theories and Phantasies, trans. Ernst Oster. Longman.
Schenker Documents Online. 2014. http://www.schenkerdocumentsonline.org
Serafine, Mary Louise, Noah Glassman, and Cornell Overbeeke. 1989. “The Cognitive Reality of Hierarchic Structure in Music.” Music Perception 6 (4): 397–430.
Smoliar, Stephen. 1980. “A Computer Aid for Schenkerian Analysis.” Computer Music Journal 4 (2): 41–59.
Sundberg, Johan, and Björn Lindblom. 1976. “Generative Theories in Language and Music Descriptions.” Cognition 4: 99–122.
Temperley, David. 2001. The Cognition of Basic Musical Structures. MIT Press.
—————. 2007. Music and Probability. MIT Press.
—————. 2008. “Hypermetrical Transitions.” Music Theory Spectrum 30 (2): 305–25.
—————. 2011. “Cognition, Perception, and Schenkerian Theory.” Music Theory Spectrum 33 (2): 146–68.
Todd, Neil. 1985. “A Model of Expressive Timing in Tonal Music.” Music Perception 3 (1): 33–57.
Wagner, Naphtali. 1995. “No Crossing Branches? The Overlapping Technique in Schenkerian Analysis.” Theory and Practice 20, 149–75.
Westergaard, Peter. 1975. An Introduction to Tonal Theory. Norton.
Yust, Jason. 2006. “Formal Models of Prolongation.” PhD diss., University of Washington.
Yust, Jason. 2009. “The Geometry of Melodic, Harmonic, and Metrical Hierarchy.” Mathematics and Computation in Music: Second International Conference: Proceedings. Springer.
http://icmpc-escom2012.web.auth.gr/sites/default/files/papers/1172_Proc.pdf
Yust, Jason. 2012. “Testing Schenkerian Theory: An Experiment on the Perception of Key Distances.” In Proceedings of the 12th International Conference on Music Perception and Cognition, 1172–1176.
http://icmpc-escom2012.web.auth.gr/sites/default/files/papers/1172_Proc.pdf
Footnotes
1. To give just a few examples, Dibben (1994) and Farbood (2012) have applied Lerdahl and Jackendoff’s theory to investigate the effects of tonal hierarchy on listener responses, Todd (1985) uses it to model the expressive timing of performers, and Temperley (2001) has applied their methods to a wide variety of problems in corpus studies.
Return to text
2. A prominent example is their distinction between grouping and metrical structure, which has been adopted by a range of authors such as Rothstein (1989) and Temperley (2008).
Return to text
3. As Temperley points out, the most promising way to undertake such a project is to derive a series of narrow testable claims from the theory, rather than attempting to take it on in some sense in toto, as the generative approach endeavors to do. Examples of studies that take this approach of testing claims of limited scope made by Schenkerian theory are Serafine, Glassman, and Overbeeke (1989); Larson (2004); and Yust (2012).
Return to text
4. The term morphology is used in roughly the same capacity in Baroni, Dalmonte, and Jacoboni 2003.
Return to text
5. Neumeyer’s sketch of a generative system for melody, however, does have one interesting non-monophonic feature in that he includes an early stage consisting of atemporal verticalized dyads. These are eventually horizontalized to create the melodic skeleton, a process that closely resembles the voice-leading transformations described below.
Return to text
6. Lerdahl and Jackendoff (1983, 273–7) themselves make the same point. Ironically, perhaps the most rhetorically charged review of the book, by Peel and Slawson, misses this entirely when they suggest that Schenker’s interpretation of the Mozart K. 331 theme could be an “alternative reading” within Lerdahl and Jackendoff’s theory (Peel and Slawson 1984, 284–5), even though Lerdahl and Jackendoff (275–7) demonstrate that Schenker’s analysis requires a realignment of bass and soprano within each measure that is not possible within their theory.
Return to text
7. Progress on this problem in regard to Lerdahl and Jackendoff (1983) has only recently been made by Hamanaka et al. (2013). Lerdahl and Jackendoff’s fusion operation (153–5) is a stopgap, but it becomes increasingly tendentious for more complex textures. See, e.g., Lerdahl’s reduction of the beginning of Beethoven’s “Waldstein” Sonata, op. 53 (2001, 37–40).
Return to text
8. Yust 2006 refers to hierarchies of musical events, like representational hierarchies, correspondingly as “static hierarchies.”
Return to text
9. Yust 2006, 52–66. “MOP” is more precise because a 2-tree can have multiple triangles originating from the same edge. However, it is also possible to have analyses consistent with the dynamic model of hierarchy where a “parent” motion has more than two “children”: i.e., instead of being made up exclusively of triangles, the “tree of edges” includes some larger polygons. These larger polygons are called “holes.” The more general class of graphs that allows for holes can be mathematically specified as “Hamiltonian outerplanar graphs” (or “HOPs”). One can imagine a HOP as a MOP with edges removed (hence, no longer maximal) as long as those edges are not on the outer perimeter (which is why the specification “Hamiltonian” is necessary). For more on the mathematical definitions and properties of these structures, see Yust 2006, 82–85, 211–13, and Yust 2009.
Return to text
10. See Yust 2006, 86–154, for further discussion of this matter.
Return to text
11. For a sequence of five events (as in the present example), there are 42 possible dynamic hierarchies (without making the assumption that the five events constitute a single motion—this assumption, which can usually be made, leaves only five possible structures). The same five events can be described by 224 representational hierarchies. These differences become more exaggerated as the number of events increases (by “combinatorial explosion”). See Yust 2006, 105–110.
Return to text
12. Larson (1997, 119–120) makes a similar point about Lerdahl’s model, and Lerdahl’s response (1997, 141–3) concedes that the model is unintuitive in certain situations (as with simple passing notes) but defends it on the grounds of simplicity and consistency. Lerdahl and Jackendoff (1983, 114–116) similarly associate dynamic hierarchy with a “network notation” that they call “impracticable.” This claim assumes that the alternative to the binary trees of events is something along the lines of the “directed acyclic graphs” described in Kirlin and Utgoff (2008). Marsden (2010) similarly dismisses directed acyclic graphs as adding a layer of computational complexity over binary trees. Dynamic hierarchy as modeled by MOPs, however, is actually considerably simpler than representational hierarchy (as shown by the combinatorial considerations in the previous note).
Return to text
13. E.g., Sundberg and Lindblöm (1976), Schachter (1980), Lerdahl and Jackendoff (1983), Longuet-Higgins and Lee (1984).
Return to text
14. It is apparently this kind of violation of hierarchical well-formedness that Lerdahl and Jackendoff have in mind when they reject the idea that passing tones and neighbor tones could be symmetrically in between the notes they elaborate—see their comments in Lerdahl and Jackendoff 1993, 308–9. It is perhaps for this reason that they assume a slippery slope from such a notion of in-betweenness to totally unrestricted free-associative networks (e.g., in Lerdahl and Jackendoff 1983, 114–116 and Lerdahl 1997; see note 12).
Return to text
15. This is not to say that the two notes (F and C) are of equivalent structural status, however, only that the structural status of F is not shown by the slur; it is shown by the beaming of F to later events and by the open notehead.
Return to text
16. The epistemological concept of synthesis was closely associated with that of the Urlinie from the beginning, however, even before Schenker’s conception of Urlinie had such specific music-theoretic content, as is in evidence in the preface of Schenker [1921] 1970 and his 1921 essay “The Urlinie: A Preliminary Remark” (2005, 21–4).
Return to text
17. Meyer’s analyses also feature the separation of melodic hierarchies into distinct voice-leading strands, like Schenker’s, and therefore represent contrapuntal hierarchies similar to the ones discussed in part 3 of this article.
Return to text
18. See, e.g., Schenker [1921–3] 2004, 32, figure 14; 60, figures 3 and 4; 75, figure 1.
Return to text
19. Schenker’s usage of the term “prolongation” is distinct from current music-theory parlance. In Counterpoint II he uses the term to refer to the ways that laws of strict counterpoint can be extended to free composition. He almost never uses the term in the modern sense of prolongation of a harmony, preferring the term Auskomponierung instead for this. See Dubiel (1990) and Yust (2006, 10–31).
Return to text
20. Lerdahl (2001, 32–34) uses this example to illustrate a related point.
Return to text
21. Discussion of these techniques constitutes a substantial portion of Part II (“The Middleground”) of Free Composition, because they are the derivations that distinguish levels of the middleground, as discussed further below.
Return to text
22. These are clearly shown with stemming in the development, and also include the
Return to text
23. Note that these figures usually begin with the Ursatz on the upper staff (except [1926] 1996, 80–81, which omits the Ursatz and first level), and the Ursatz is not related to level one by transformation. To abbreviate the top-down portion of his analyses and avoid delaying his discussion of the foreground, which occupies the bulk of his analytical writing, Schenker generally limits his middleground derivations to two or three levels. Because this seems to be a practical necessity for writing a compelling analytical essay, it should not be taken to imply that additional levels of derivation do not exist. On structural levels and the distinction between middleground and foreground, see Proctor and Riggins (1988).
Return to text
24. A typical example of changing species is the harmonization of a passing tone, which involves a switch from a second-species setting involving a dissonant passing tone to a consonant note-against-note setting. These are to be understood not as simple second and first species, however, but as mixed species with an imaginary cantus firmus (Schenker [1922] 1987, 181–5).
Return to text
25. The publication date of these analyses does not always match the date of their conception, an obvious instance being the analysis of Beethoven’s Fifth Symphony, which is spread over three volumes of Tonwille (I, V, and VI) because of its length (see Bent and Drabkin’s preface to Schenker [1921–3] 2004, v–viii). A telling example is the analysis of the first movement of Beethoven’s Piano Sonata op. 10 no. 2 in “On Organicism in Sonata Form” ([1926] 1996, 25–28). According to Schenker’s diary, he worked on an analysis of the piece much earlier, in March of 1924 (Schenker Documents Online, OJ 3/6, page 23, transcr. Deisinger, trans. Ferguson); however, he incorporates it into an essay written after a shift in his theory of linear progression (see below). Therefore the analytical graph reflects an earlier practice of Stimmführungsprolongationen, highlighting prolongations of counterpoint (such as harmonization of a passing tone) and treating motion to an inner voice as a prolongation. Yet the text accompanying the graph ties the analysis to Schenker’s newer theory of linear progression, highlighting the retention of the head tone and the generation of content out of the linear progressions.
Return to text
26. This is especially evident in, e.g., the analysis of Beethoven’s Fifth Symphony (Schenker [1921–3] 2004, 30) where he refers to a diminished fourth in the Urlinie as a fourth-progression, and in the analysis of Mozart’s Piano Sonata K. 310 (Schenker [1921–3] 2004, 55), where he refers to motivic diminished fifths as fifth-progressions.
Return to text
27. See “Composing Out by Linear Progressions” in “Further Considerations of the Urlinie: I” (Schenker [1925] 1994, 107–109), “Further Considerations of the Urlinie: II” (Schenker [1926] 1996, 1–19) and §205–207 of Free Composition (Schenker [1935] 1979, 74–75).
Return to text
28. See the analyses of Schubert’s Impromptu no. 3, D. 899 and Mendelssohn’s “Song without Words” op. 67 no. 6 (Schenker [1923–4] 2005, 137–42, 150–3).
Return to text
29. Schenker [1925] 1994, 104–111; [1926] 1996, 1–19.
Return to text
30. Schenker’s diary indicates that he had analyzed this first movement much earlier, in May of 1923 (Schenker Documents Online, OJ 3/4, page 30 and OJ 3/4, page 29, transcr. Deisinger, trans. Ferguson) and before that in 1922 (Schenker Documents Online, OJ 3/4, page 10 and pages 18–19, transcr. Deisinger, trans. Witmer). The published analysis was completed relatively early, in January of 1924 (Schenker Documents Online, OJ 3/6, page 27, transcr. Deisinger, trans. Ferguson).
Return to text
31. Schenker’s footnote to the analysis of Bach’s Little Prelude no. 3 (2004, 175) is particularly telling: he calls the motion to an inner voice a “prolonged form of a third-progression,” meaning that it is a free imitation (prolongation) of a more fundamental, more strict, contrapuntal phenomenon (third-progression)—in short, it is not a true third-progression. On Schenker’s usage of the term “prolongation,” see Dubiel (1990) and Yust (2006, 10–31).
Return to text
32. Schenker cites this passage as an example of “boundary play” (§143), perhaps in allusion to his interpretation of the Gs.
Return to text
33. As does Neumeyer (1987), who makes similar observations about the piece, but considers G to be an ascending passing tone rather than an upper neighbor. According to his interpretation, the G and A at the end of m. 7 are successive notes in a single voice, even though they both are sustained as part of the dominant ninth harmony over all of mm. 5–7.
Return to text
34. Note that the first counterpoint to the Ursatz appears at the same level, level 1, in accordance with the convention that voice-leading transformations distinguish levels, not the addition of new voices. Also, level 2 is what Schenker would typically call the “first level,” because he does not include the Ursatz in his numbering.
Return to text
35. The reader may be disturbed by the fact that the secondary upper voice added at level 1 moves in parallel fifths with the Urlinie: G–F / C–
Return to text
Copyright Statement
Copyright © 2015 by the Society for Music Theory. All rights reserved.
[1] Copyrights for individual items published in Music Theory Online (MTO) are held by their authors. Items appearing in MTO may be saved and stored in electronic or paper form, and may be shared among individuals for purposes of scholarly research or discussion, but may not be republished in any form, electronic or print, without prior, written permission from the author(s), and advance notification of the editors of MTO.
[2] Any redistributed form of items published in MTO must include the following information in a form appropriate to the medium in which the items are to appear:
This item appeared in Music Theory Online in [VOLUME #, ISSUE #] on [DAY/MONTH/YEAR]. It was authored by [FULL NAME, EMAIL ADDRESS], with whose written permission it is reprinted here.
[3] Libraries may archive issues of MTO in electronic or paper form for public access so long as each issue is stored in its entirety, and no access fee is charged. Exceptions to these requirements must be approved in writing by the editors of MTO, who will act in accordance with the decisions of the Society for Music Theory.
This document and all portions thereof are protected by U.S. and international copyright laws. Material contained herein may be copied and/or distributed for research purposes only.
Prepared by Rebecca Flore, Editorial Assistant
Number of visits:
15012